目录

例一:LeetCode70



/**
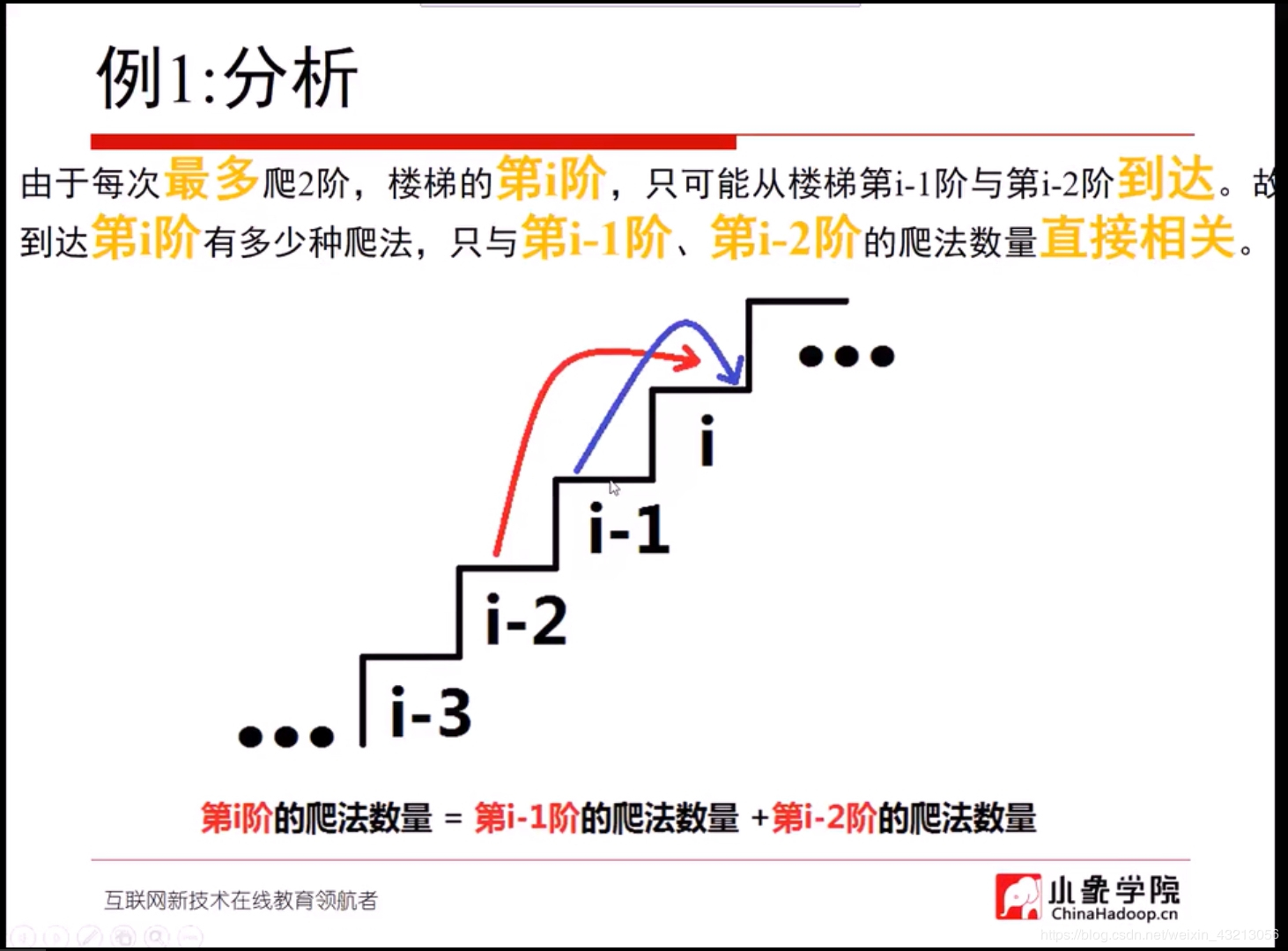
You are climbing a stair case. It takes n steps to reach to the top.
Each time you can either climb 1 or 2 steps. In how many distinct ways can you climb to the top?
*/
#include <stdio.h>
#include <vector>
class Solution {
public:
int climbStairs(int n) {
std::vector<int> dp(n + 3, 0); // 创建长度为n + 3的数组,初始化为0
dp[1] = 1;
dp[2] = 2;
for (int i = 3; i <= n; i++) {
dp[i] = dp[i - 1] + dp[i - 2];
}
return dp[n];
}
};
int main() {
Solution solve;
printf("%d\n", solve.climbStairs(3));
return 0;
}

例二:LeetCode198




/**
You are a professional robber planning to rob houses along a street.
Each house has a certain amount of money stashed, the only constraint
stopping you from robbing each of them is that adjacent houses have
security system connected and it will automatically contact the police
if two adjacent houses were broken into on the same night.
Given a list of non-negative integers representing the amount of money
of each house, determine the maximum amount of money you can rob tonight
without alerting the police.
*/
#include <stdio.h>
#include <vector>
class Solution {
public:
int rob(std::vector<int>& nums) {
if (nums.size() == 0) {
return 0;
}
if (nums.size() == 1) {
return nums[0];
}
std::vector<int> dp(nums.size(), 0);
dp[0] = nums[0];
dp[1] = std::max(nums[0], nums[1]);
for (int i = 2; i < nums.size(); i++) {
dp[i] = std::max(dp[i - 1], nums[i] + dp[i - 2]);
}
return dp[nums.size() - 1];
}
};
int main() {
Solution solve;
std::vector<int> nums;
nums.push_back(5);
nums.push_back(2);
nums.push_back(6);
nums.push_back(3);
nums.push_back(1);
nums.push_back(7);
printf("%d\n", solve.rob(nums));
return 0;
}
例三:LeetCode53



/**
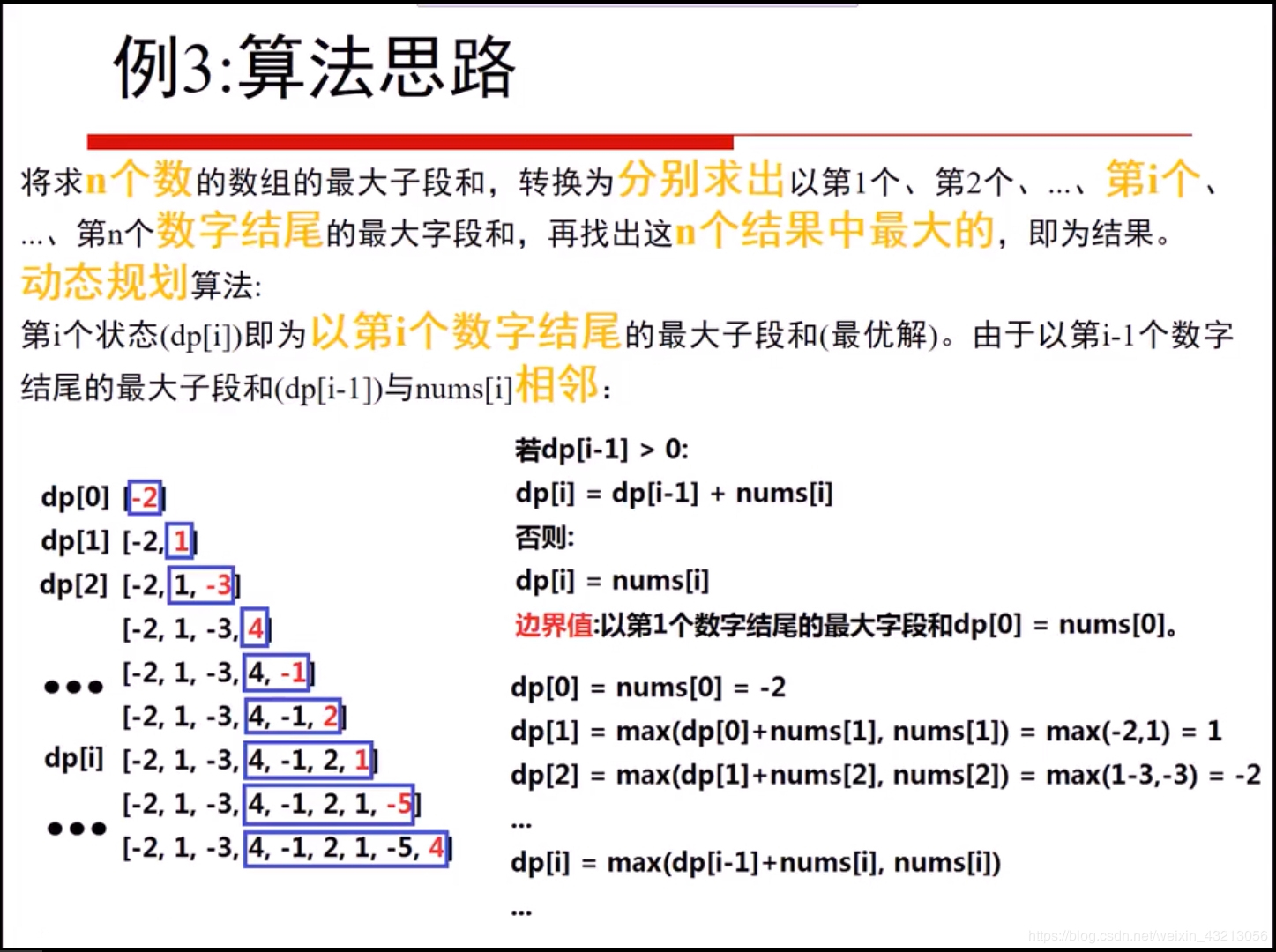
Given an integer array nums, find the contiguous subarray
(containing at least one number) which has the largest sum and return its sum.
*/
#include <stdio.h>
#include <vector>
class Solution {
public:
int maxSubArray(std::vector<int>& nums) {
std::vector<int> dp(nums.size(), 0);
dp[0] = nums[0];
int max_res = dp[0];
for (int i = 1; i < nums.size(); i++) {
dp[i] = std::max(dp[i - 1] + nums[i], nums[i]);
if (dp[i] > max_res) {
max_res = dp[i];
}
}
return max_res;
}
};
int main() {
Solution solve;
std::vector<int> nums;
nums.push_back(-2);
nums.push_back(1);
nums.push_back(-3);
nums.push_back(4);
nums.push_back(-1);
nums.push_back(2);
nums.push_back(1);
nums.push_back(-5);
nums.push_back(4);
printf("%d\n", solve.maxSubArray(nums));
return 0;
}
例四:LeetCode32


/**
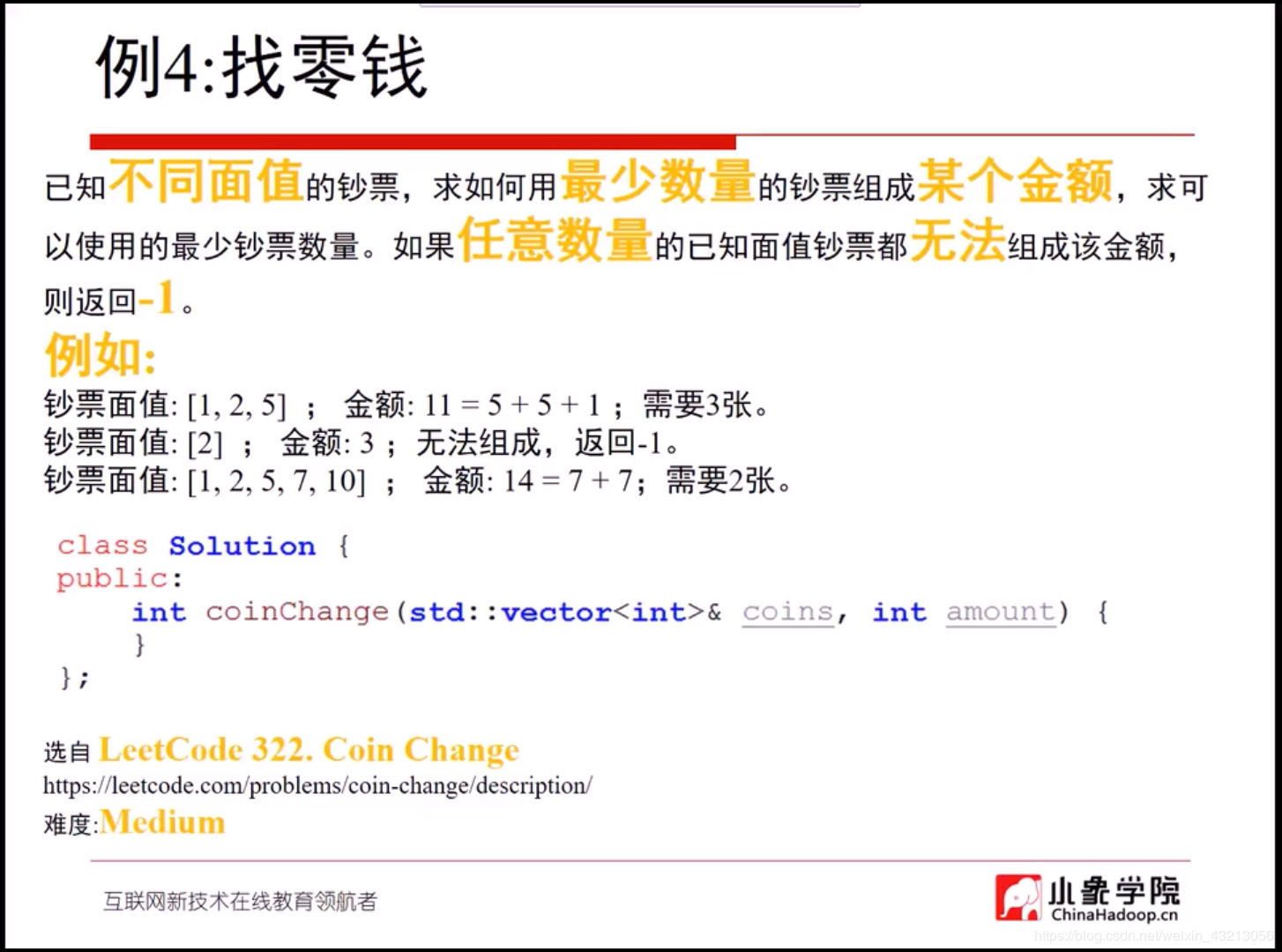
You are given coins of different denominations and a total amount of money amount.
Write a function to compute the fewest number of coins that you need to make up
that amount. If that amount of money cannot be made up by any combination of the
coins, return -1.
*/
#include <stdio.h>
#include <vector>
class Solution {
public:
int coinChange(std::vector<int>& coins, int amount) {
std::vector<int> dp;
for (int i = 0; i <= amount; i++) {
dp.push_back(-1);
}
dp[0] = 0;
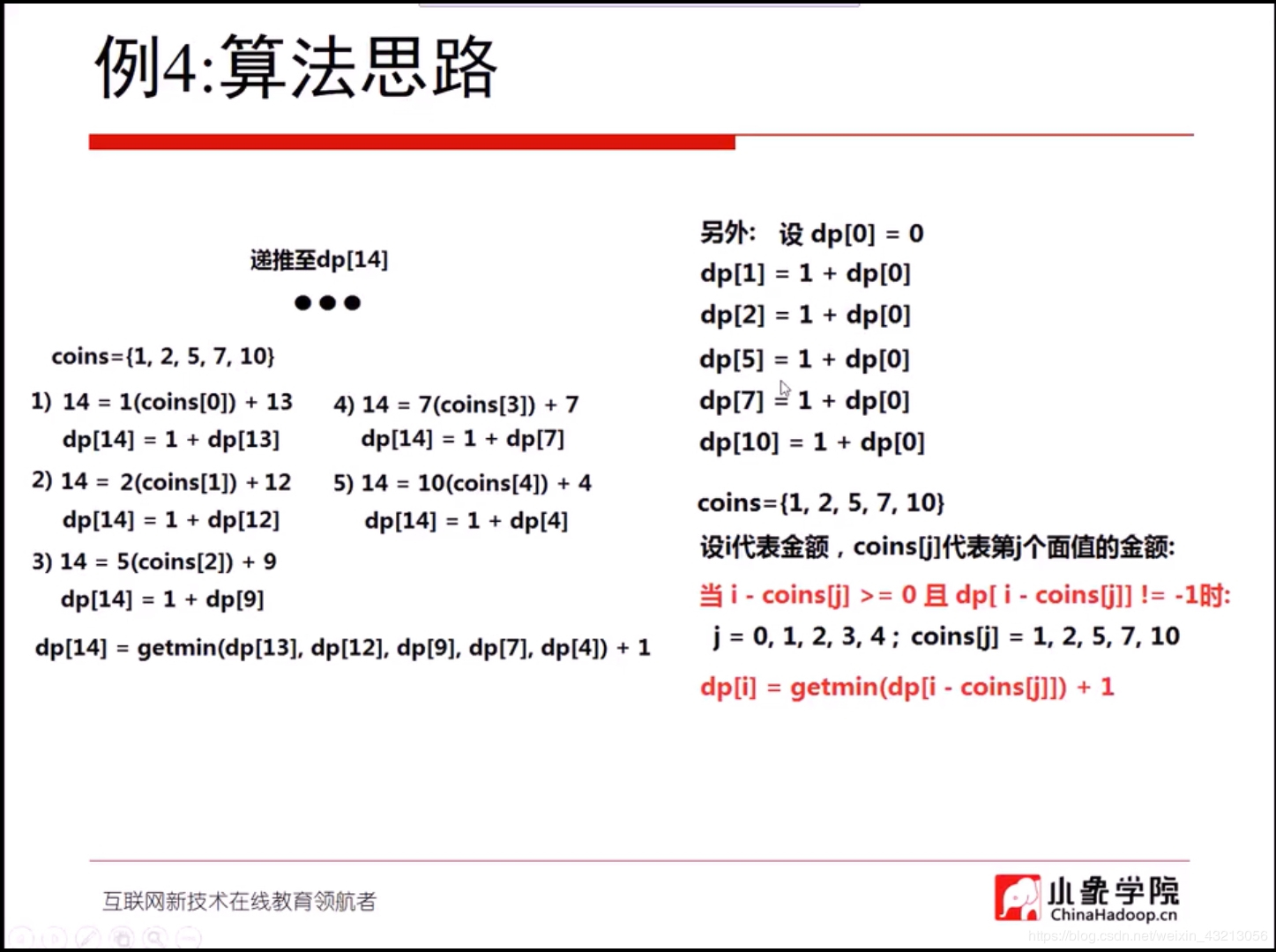
for (int i = 1; i <= amount; i++) {
for (int j = 0; j < coins.size(); j++) {
if (i - coins[j] >= 0 && dp[i - coins[j]] != -1) {
if (dp[i] == -1 || dp[i] > dp[i - coins[j]] + 1) {
dp[i] = dp[i - coins[j]] + 1;
}
}
}
}
return dp[amount];
}
};
int main() {
Solution solve;
std::vector<int> coins;
coins.push_back(1);
coins.push_back(2);
coins.push_back(5);
coins.push_back(7);
coins.push_back(10);
for (int i = 1; i <= 14; i++) {
printf("dp[%d] = %d\n", i, solve.coinChange(coins, i));
}
return 0;
}
例五:LeetCode120

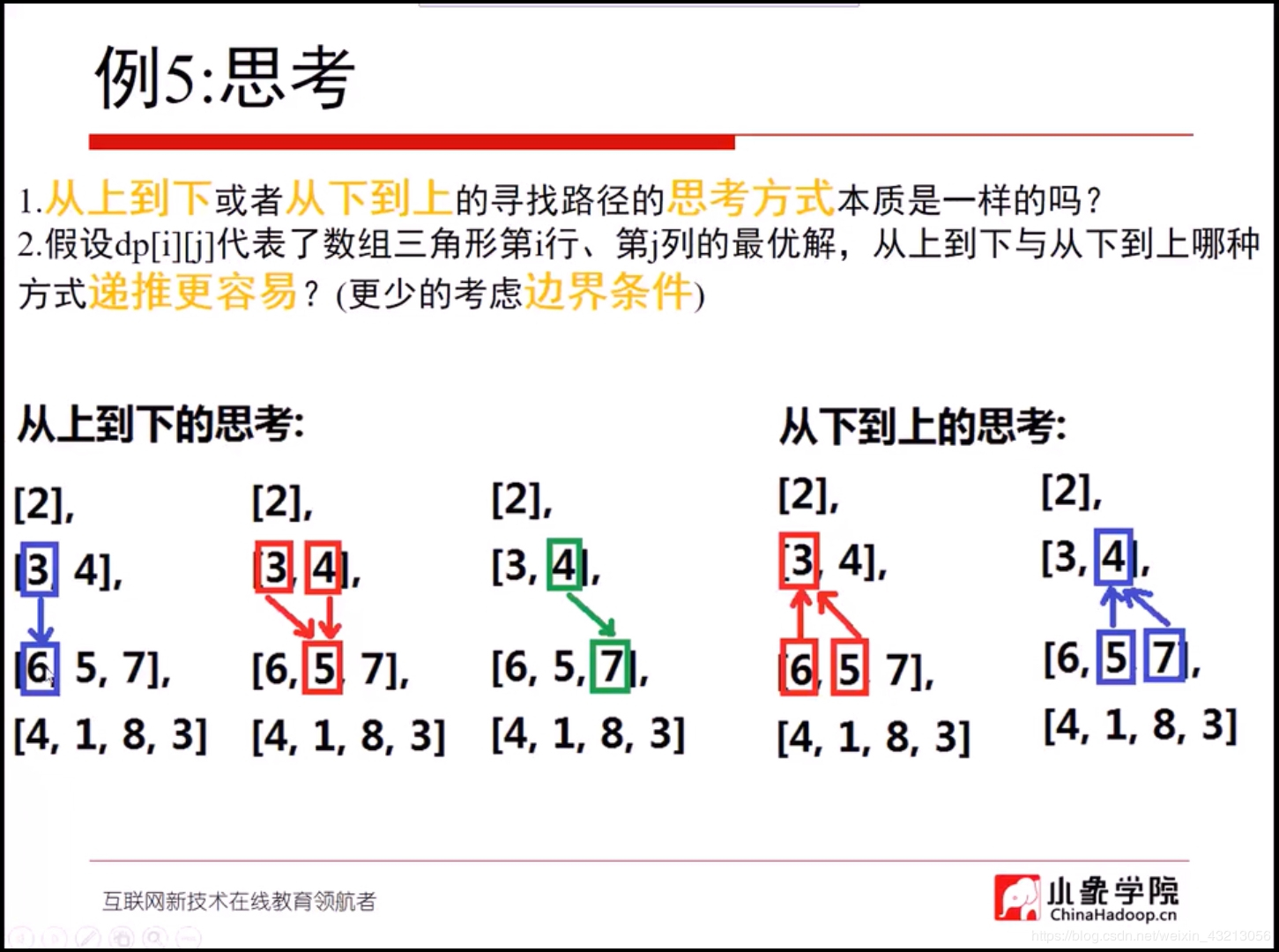
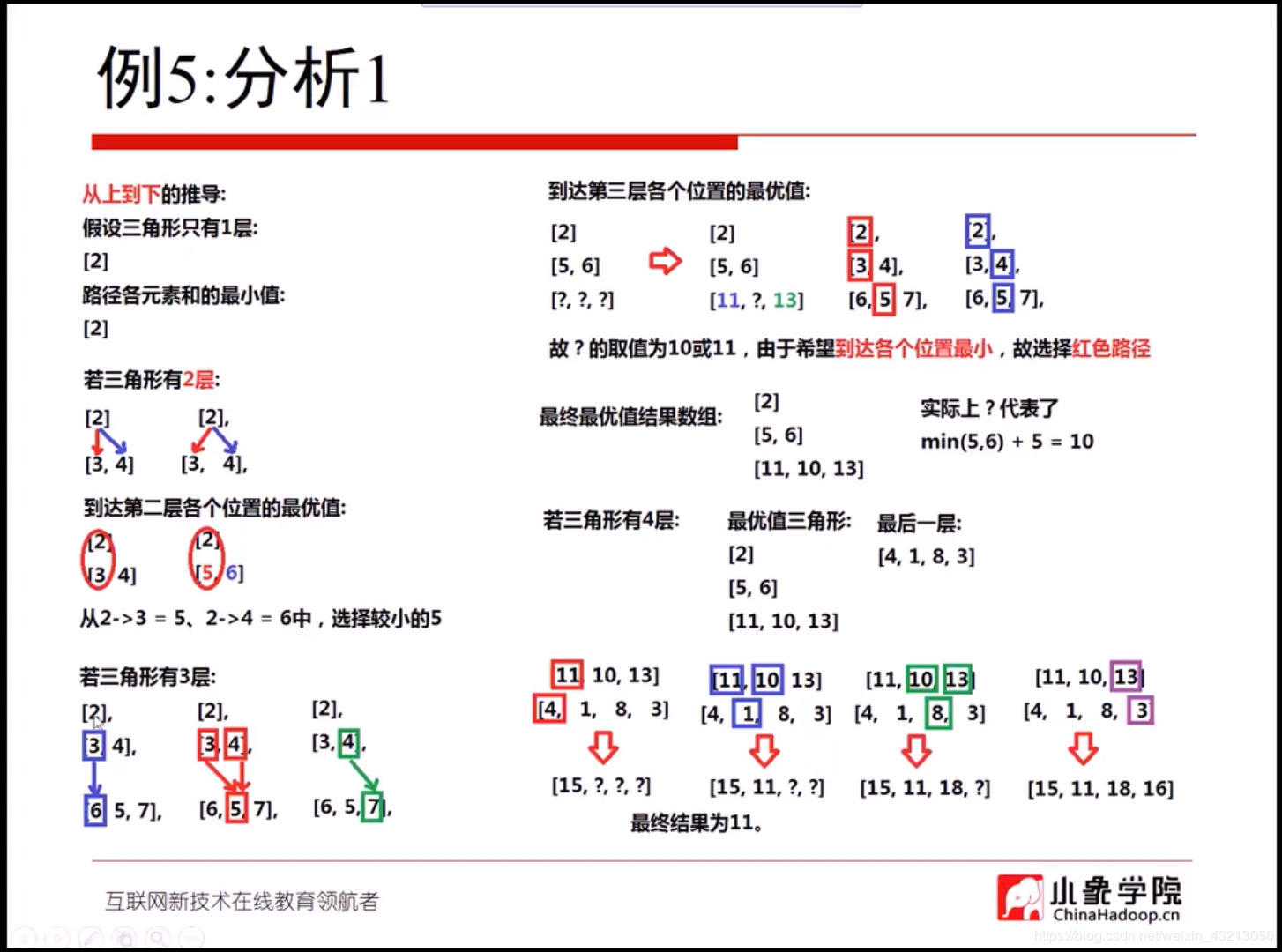


/**
Given a triangle, find the minimum path sum from top to bottom.
Each step you may move to adjacent numbers on the row below.
For example, given the following triangle
*/
#include <stdio.h>
#include <vector>
class Solution {
public:
int minimumTotal(std::vector<std::vector<int>>& triangle) {
if (triangle.size() == 0) {
return 0;
}
std::vector<std::vector<int>> dp;
for (int i = 0; i < triangle.size(); i++) {
dp.push_back(std::vector<int>());
for (int j = 0; j < triangle[i].size(); j++) {
dp[i].push_back(0);
}
}
for (int i = 0; i < dp.size(); i++) {
dp[dp.size() - 1][i] = triangle[dp.size() - 1][i];
}
for (int i = dp.size() - 2; i >= 0; i--) {
for (int j = 0; j < dp[i].size(); j++) {
dp[i][j] = std::min(dp[i + 1][j], dp[i + 1][j + 1]) + triangle[i][j];
}
}
return dp[0][0];
}
};
int main() {
std::vector<std::vector<int>> triangle;
int test[][10] = { {2}, {3, 4}, {6, 5, 7}, {4, 1, 8, 3} };
for (int i = 0; i < 4; i++) {
triangle.push_back(std::vector<int>());
for (int j = 0; j < i + 1; j++) {
triangle[i].push_back(test[i][j]);
}
}
Solution solve;
printf("%d\n", solve.minimumTotal(triangle));
return 0;
}
例六:LeetCode300




class Solution {
public:
int lengthOfLIS(std::vector<int>& nums) {
if (nums.size() == 0) {
return 0;
}
std::vector<int> dp(nums.size(), 0);
dp[0] = 1;
int LIS = 1;
for (int i = 1; i < dp.size(); i++) {
dp[i] = 1;
for (int j = 0; j < i; j++) {
if (nums[i] > nums[j] && dp[i] < dp[j] + 1) {
dp[i] = dp[j] + 1;
}
}
if (LIS < dp[i]) {
LIS = dp[i];
}
}
return LIS;
}
};
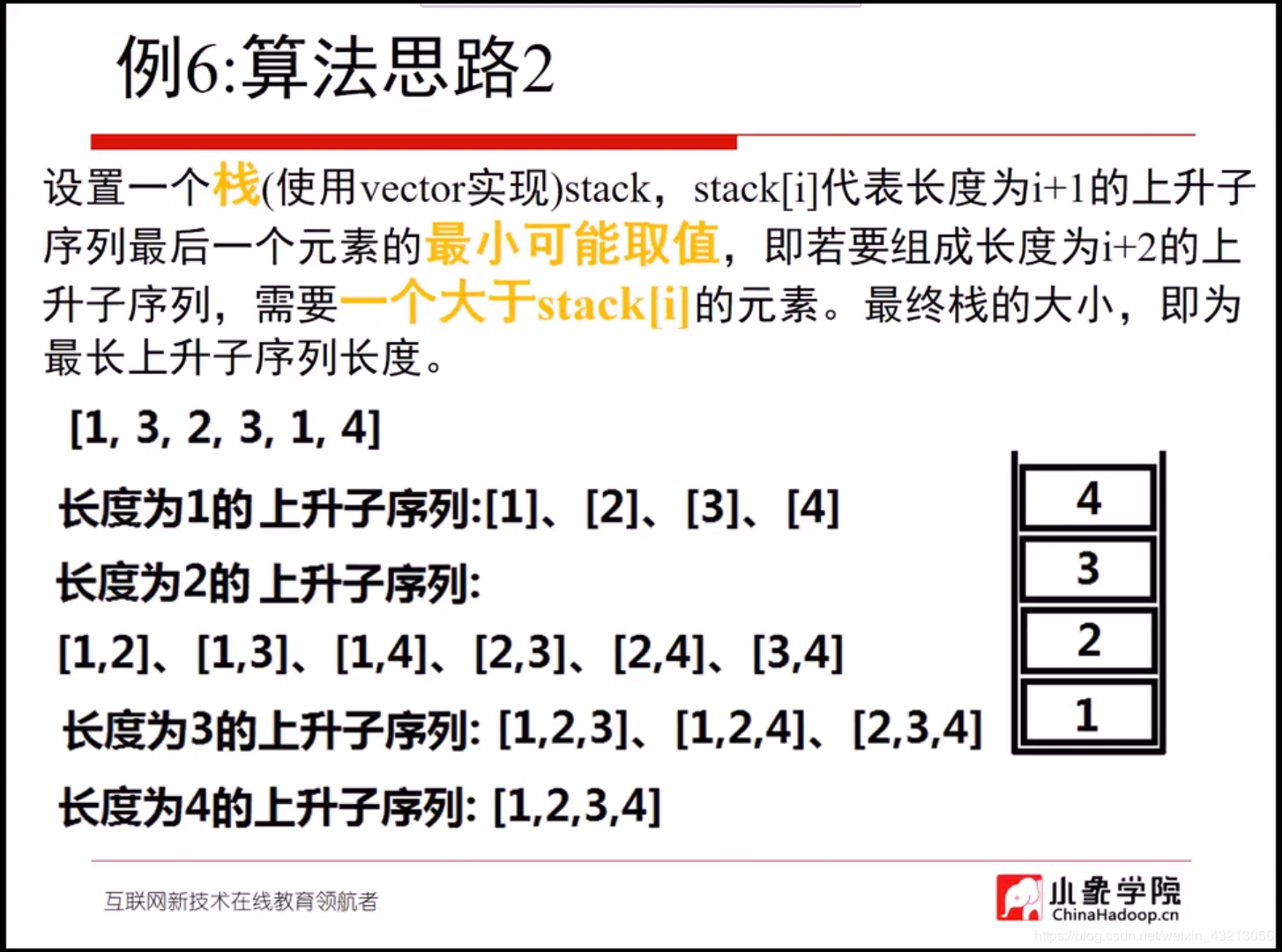
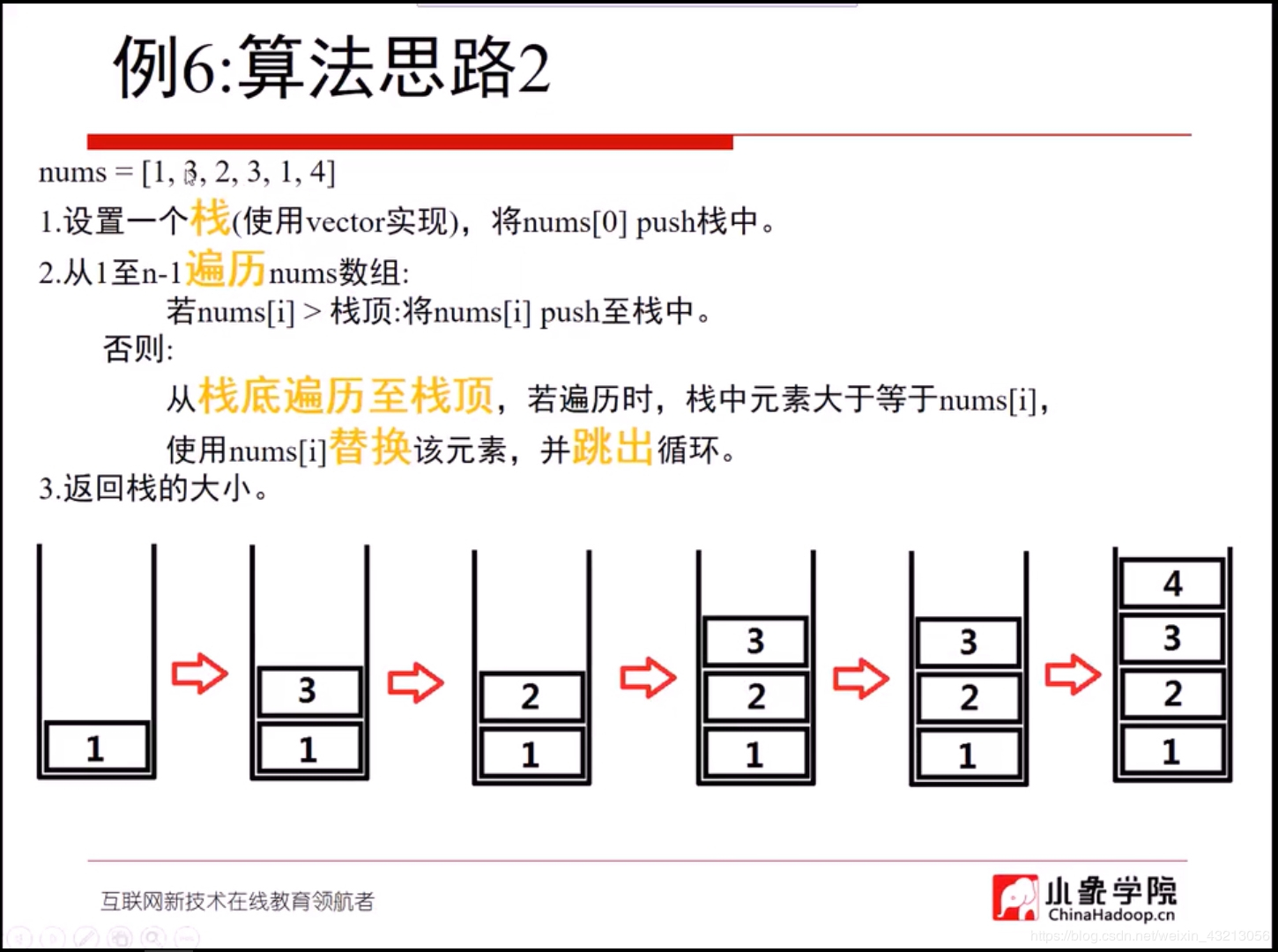
/**
Given an unsorted array of integers, find the length of longest increasing subsequence.
*/
#include <stdio.h>
#include <vector>
// // O(n^2)
// class Solution{
// public:
// int lengthOfLIS(std::vector<int> &nums){
// if(nums.size() == 0){
// return 0;
// }
// std::vector<int> dp(nums.size(), 0);
// dp[0] = 1;
// int LIS = 1;
// for(int i = 1; i < dp.size(); i++){
// dp[i] = 1;
// for(int j = 0; j < i; j++){
// if(nums[i] > nums[j] && dp[i] < dp[j] + 1){
// dp[i] = dp[j] + 1;
// }
// }
// if(LIS < dp[i]){
// LIS = dp[i];
// }
// }
// return LIS;
// }
// };
// 更优算法 用二分法优化查找后时间复杂度为 n*logn
class Solution {
public:
int lengthOfLIS(std::vector<int>& nums) {
if (nums.size() == 0) {
return 0;
}
std::vector<int> stack;
stack.push_back(nums[0]);
for (int i = 1; i < nums.size(); i++) {
if (nums[i] > stack.back()) { // 返回stack中的最后一个元素
stack.push_back(nums[i]);
}
else {
// for(int j = 0; j < stack.size(); j++){
// if(stack[j] >= nums[i]){
// stack[j] = nums[i];
// break;
int pos = binary_search(stack, nums[i]); // logn
stack[pos] = nums[i];
}
}
return stack.size();
}
private:
int binary_search(std::vector<int> nums, int target) {
int index = -1;
int begin = 0;
int end = nums.size() - 1;
while (index == -1) {
int mid = (begin + end) / 2;
if (target == nums[mid]) {
index = mid;
}
else if (target < nums[mid]) {
if (mid == 0 || target > nums[mid - 1]) {
index = mid;
}
end = mid - 1;
}
else if (target > nums[mid]) {
if (mid == nums.size() - 1 || target < nums[mid + 1]) {
index = mid + 1;
}
begin = mid + 1;
}
}
return index;
}
};
int main() {
int test[] = { 1, 3, 2, 3, 1, 4 };
std::vector<int> nums;
for (int i = 0; i < 6; i++) {
nums.push_back(test[i]);
}
Solution solve;
printf("%d\n", solve.lengthOfLIS(nums));
return 0;
}
例七:LeetCode64
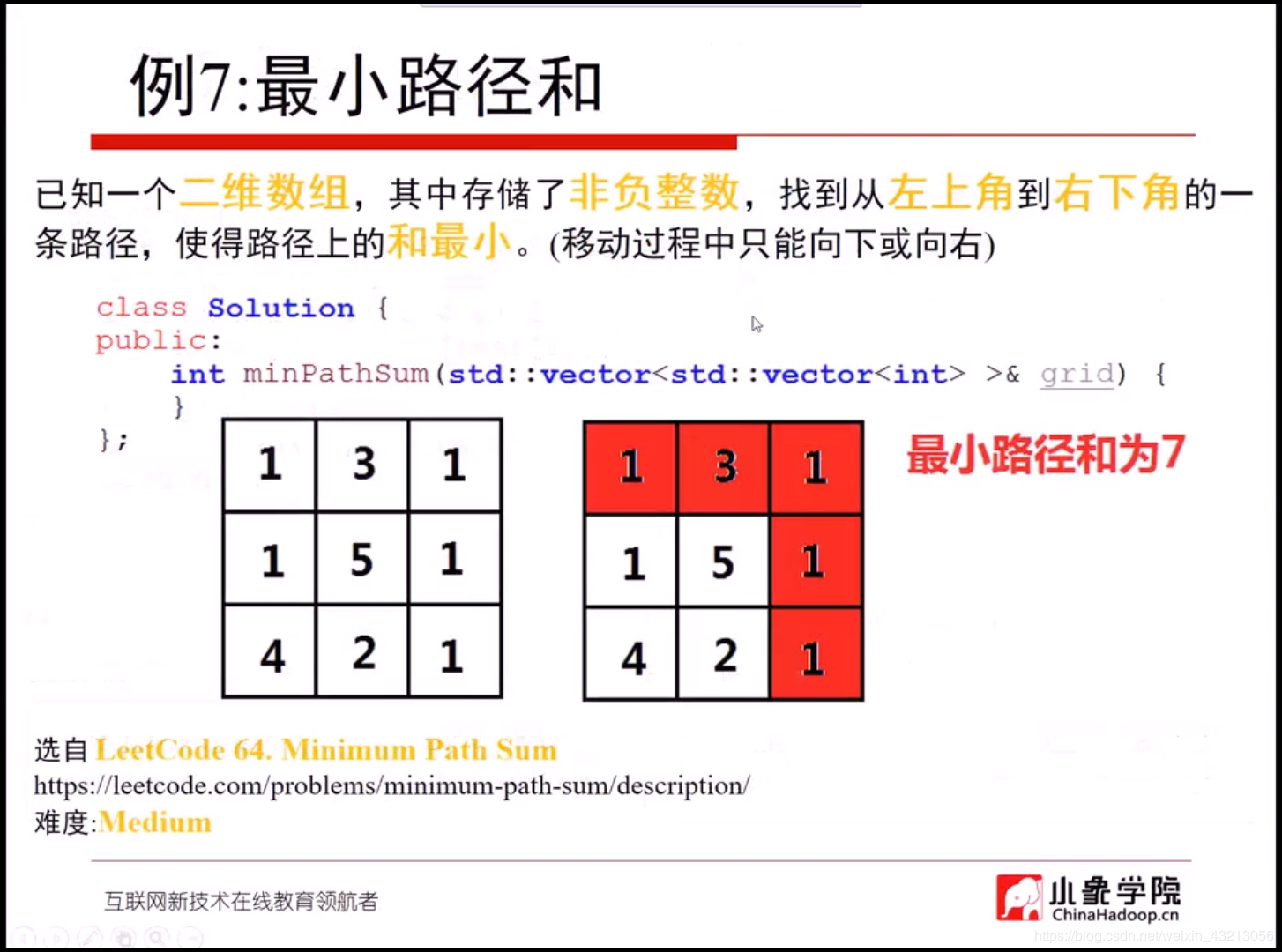
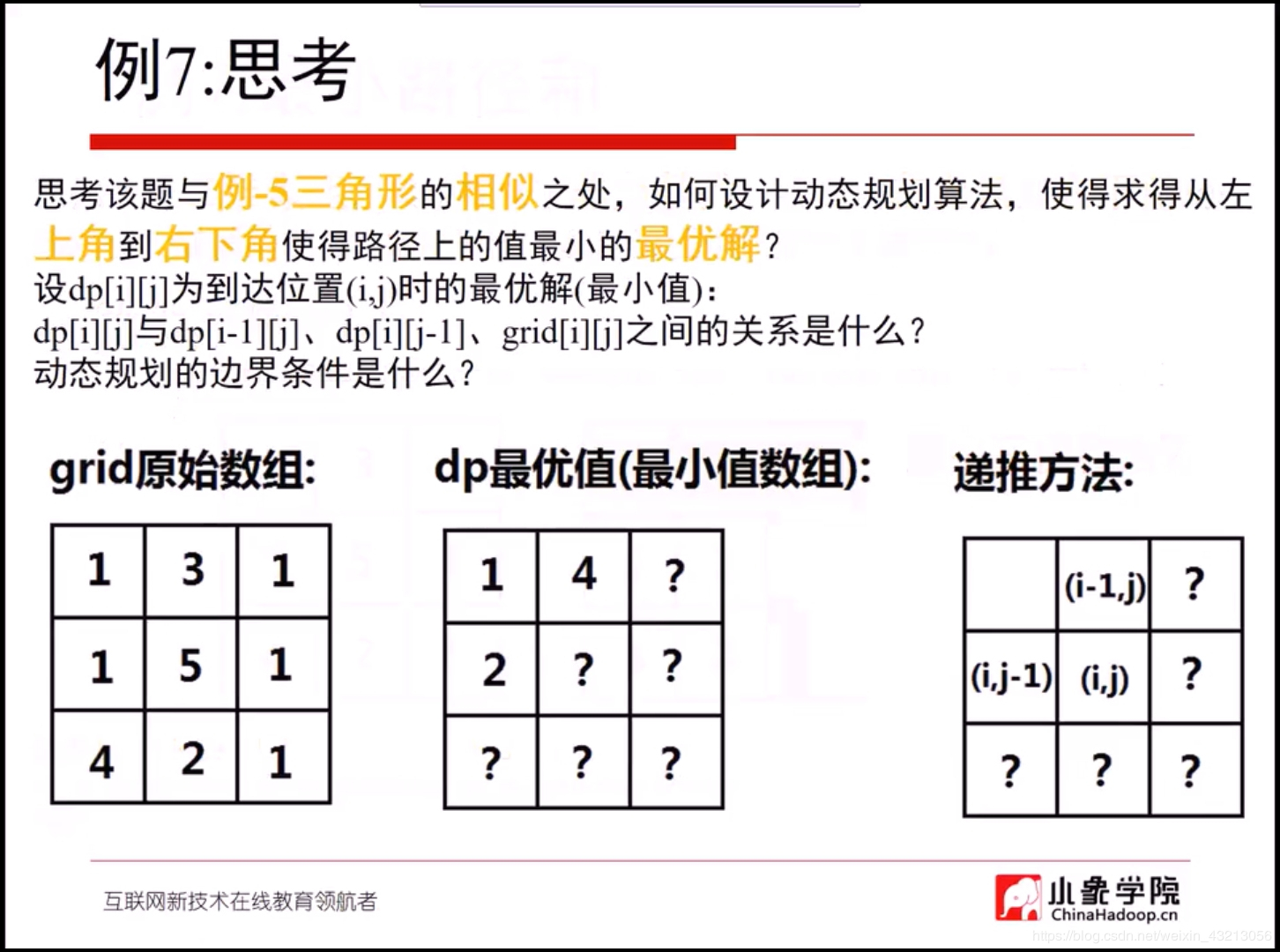
/**
Given a m x n grid filled with non-negative numbers,
find a path from top left to bottom right which minimizes the sum of all numbers along its path.
*/
#include <stdio.h>
#include <vector>
class Solution {
public:
int minPathSum(std::vector<std::vector<int>>& grid) {
if (grid.size() == 0) {
return 0;
}
int row = grid.size();
int column = grid[0].size();
std::vector<std::vector<int>> dp(row, std::vector<int>(column, 0));
dp[0][0] = grid[0][0];
for (int i = 1; i < column; i++) {
dp[0][i] = dp[0][i - 1] + grid[0][i];
}
for (int i = 1; i < row; i++) {
dp[i][0] = dp[i - 1][0] + grid[i][0];
for (int j = 1; j < column; j++) {
dp[i][j] = std::min(dp[i - 1][j], dp[i][j - 1]) + grid[i][j];
}
}
return dp[row - 1][column - 1];
}
};
int main() {
int test[][3] = { {1, 3, 1}, {1, 5, 1}, {4, 2, 1} };
std::vector<std::vector<int>> grid;
for (int i = 0; i < 3; i++) {
grid.push_back(std::vector<int>());
for (int j = 0; j < 3; j++) {
grid[i].push_back(test[i][j]);
}
}
Solution solve;
printf("%d\n", solve.minPathSum(grid));
return 0;
}
例八:LeetCode174



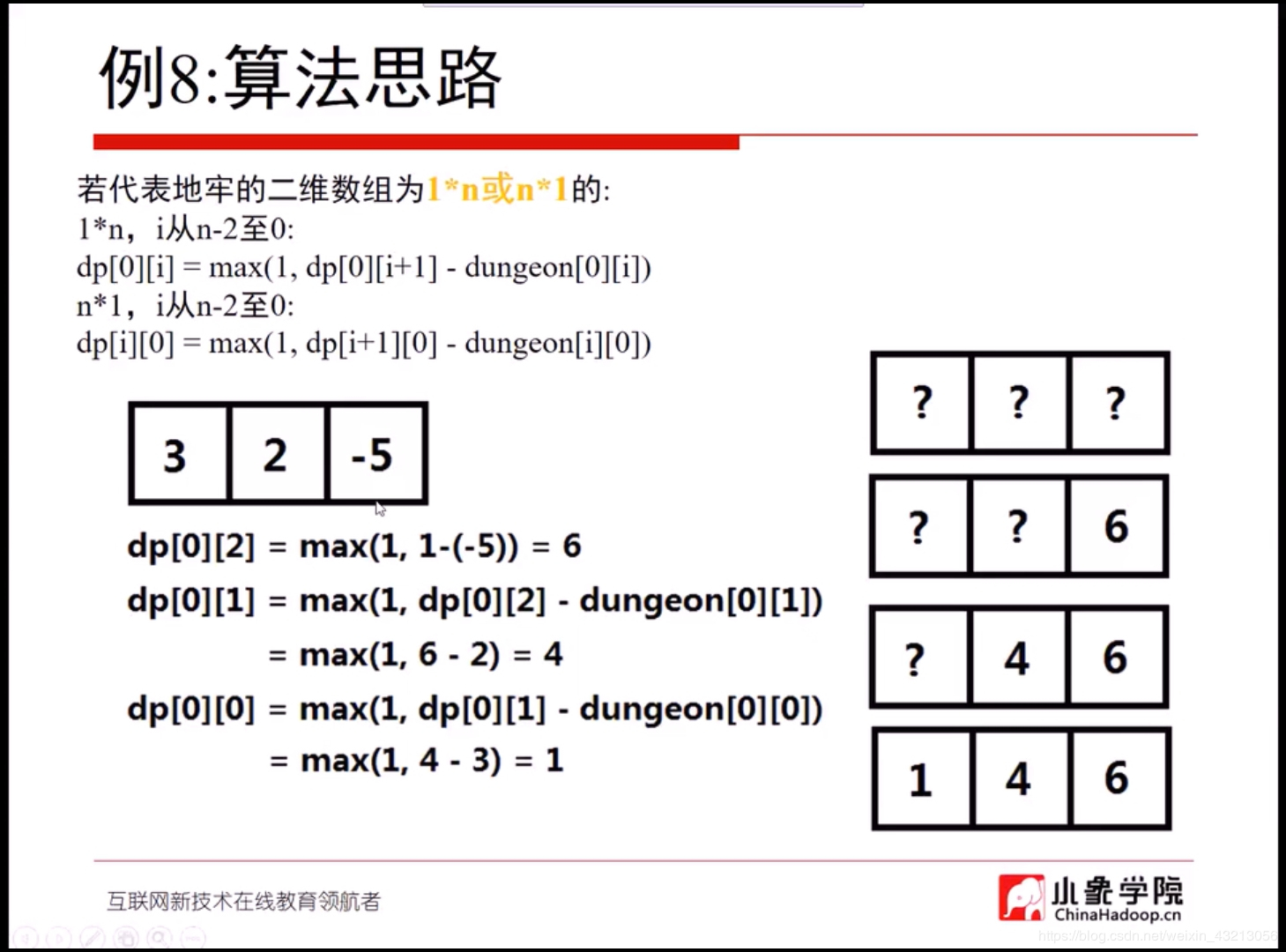
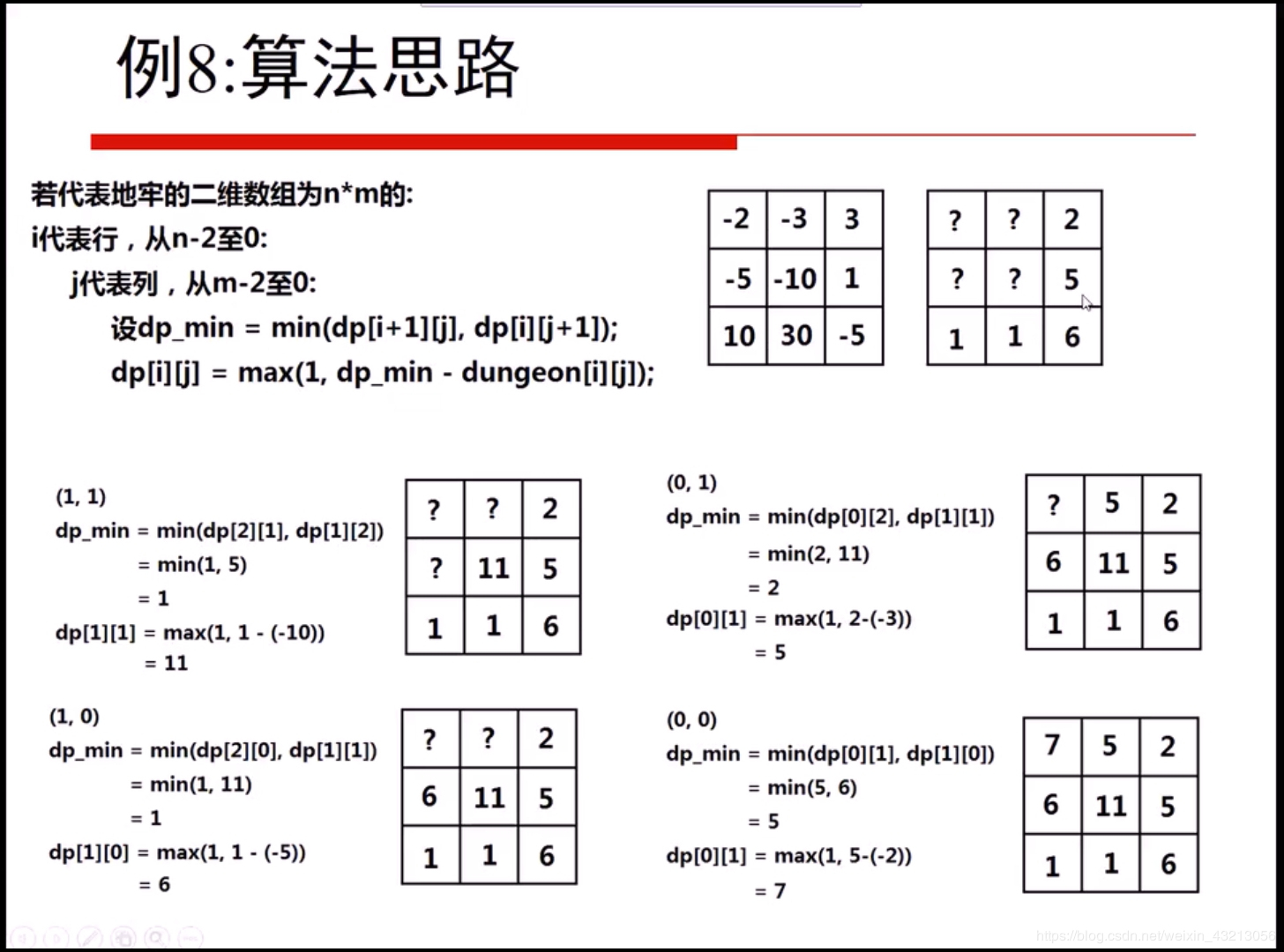
c /** The demons had captured the princess (P) and imprisoned her in the bottom-right corner of a dungeon. The dungeon consists of M x N rooms laid out in a 2D grid. Our valiant knight (K) was initially positioned in the top-left room and must fight his way through the dungeon to rescue the princess. The knight has an initial health point represented by a positive integer. If at any point his health point drops to 0 or below, he dies immediately. Some of the rooms are guarded by demons, so the knight loses health (negative integers) upon entering these rooms; other rooms are either empty (0's) or contain magic orbs that increase the knight's health (positive integers). In order to reach the princess as quickly as possible, the knight decides to move only rightward or downward in each step. */ #include <stdio.h> #include <vector> class Solution { public: int calculateMinimumHP(std::vector<std::vector<int>>& dungeon) { if (dungeon.size() == 0) { return 0; } std::vector<std::vector<int>> dp(dungeon.size(), std::vector<int>(dungeon[0].size(), 0)); int row = dungeon.size(); int column = dungeon[0].size(); dp[row - 1][column - 1] = std::max(1, 1 - dungeon[row - 1][column - 1]); for (int i = column - 2; i >= 0; i--) { dp[row - 1][i] = std::max(1, dp[row - 1][i + 1] - dungeon[row - 1][i]); } for (int i = row - 2; i >= 0; i--) { dp[i][column - 1] = std::max(1, dp[i + 1][column - 1] - dungeon[i][column - 1]); } for (int i = row - 2; i >= 0; i--) { for (int j = column - 2; j >= 0; j--) { int dp_min = std::min(dp[i + 1][j], dp[i][j + 1]); dp[i][j] = std::max(1, dp_min - dungeon[i][j]); } } return dp[0][0]; } }; int main() { int test[][3] = { {-2, -3, 3}, {-5, -10, 1}, {10, 30, -5} }; std::vector<std::vector<int>> dungeon; for (int i = 0; i < 3; i++) { dungeon.push_back(std::vector<int>()); for (int j = 0; j < 3; j++) { dungeon[i].push_back(test[i][j]); } } Solution solve; printf("%d\n", solve.calculateMinimumHP(dungeon)); return 0; }