模糊控制
一、代码实现
二、结果展示
三、总结
一、代码实现
a=newfis('fuzzf');
f1=2;
a=addvar(a,'input','e',[-2*f1,3*f1]);
a=addmf(a,'input',1,'NB','zmf',[-2*f1,2*f1]);
a=addmf(a,'input',1,'NM','trimf',[-2*f1,-2*f1,0]);
a=addmf(a,'input',1,'NS','trimf',[-2*f1,-1*f1,1*f1]);
a=addmf(a,'input',1,'Z','trimf',[-3*f1,0,2*f1]);
a=addmf(a,'input',1,'PS','trimf',[-1*f1,1*f1,3*f1]);
a=addmf(a,'input',1,'PM','trimf',[0,2*f1,3*f1]);
a=addmf(a,'input',1,'PB','smf',[1*f1,3*f1]);
f2=2;
a=addvar(a,'input','ec',[-3*f2,4*f2]); a=addmf(a,'input',2,'NB','zmf',[-3*f2,-1*f2]);
a=addmf(a,'input',2,'NM','trimf',[-2*f2,-1*f2,0]);
a=addmf(a,'input',2,'NS','trimf',[-3*f2,-1*f2,1*f2]);
a=addmf(a,'input',2,'Z','trimf',[-2*f2,1,2*f2]);
a=addmf(a,'input',2,'PS','trimf',[-1*f2,1*f2,2*f2]);
a=addmf(a,'input',2,'PM','trimf',[0,1*f2,2*f2]);
a=addmf(a,'input',2,'PB','smf',[1*f2,2*f2]);
f3=2;
a=addvar(a,'output','u',[-3*f3,3*f3]);
a=addmf(a,'output',1,'NB','zmf',[-2*f3,-1*f3]);
a=addmf(a,'output',1,'NM','trimf',[-2*f3,0,1*f3]);
a=addmf(a,'output',1,'NS','trimf',[-3*f3,1,1*f3]);
a=addmf(a,'output',1,'Z','trimf',[-1*f3,0,2*f3]);
a=addmf(a,'output',1,'PS','trimf',[-1*f3,1*f3,2*f3]);
a=addmf(a,'output',1,'PM','trimf',[0,1*f3,2*f3]);
a=addmf(a,'output',1,'PB','smf',[1*f3,2*f3]);
rulelist=[1 2 1 1 1;
1 2 1 1 1;
1 3 3 1 1;
1 4 2 1 1;
1 5 2 1 1;
1 6 4 1 1;
1 7 4 1 1;
2 2 2 1 1;
2 2 2 1 1;
2 3 3 1 1;
2 4 2 1 1;
2 5 3 1 1;
2 6 2 1 1;
2 7 5 1 1;
3 2 1 1 1;
3 2 2 1 1;
3 3 2 1 1;
3 4 2 1 1;
3 5 3 1 1;
3 6 4 1 1;
3 7 5 1 1;
];
a=addrule(a,rulelist);
showrule(a) a1=setfis(a,'DefuzzMethod','centroid');
writefis(a1,'fuzzf');
a2=readfis('fuzzf');
disp('fuzzy Controller table:e=[-3,+3],ec=[-3,+3]');
Ulist=zeros(7,7);
for i=1:7
for j=1:7
e(i)=-4+i;
ec(j)=-4+j;
Ulist(i,j)=evalfis([e(i),ec(j)],a2);
end
end
Ulist
figure(1); plotfis(a2);
figure(2);plotmf(a,'input',1);
figure(3);plotmf(a,'input',2);
figure(4);plotmf(a,'output',1);
二、结果展示
e的隶属度函数
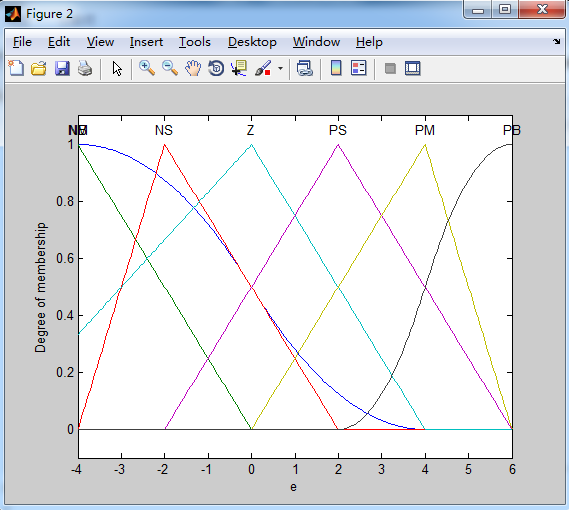
ec的隶属度函数
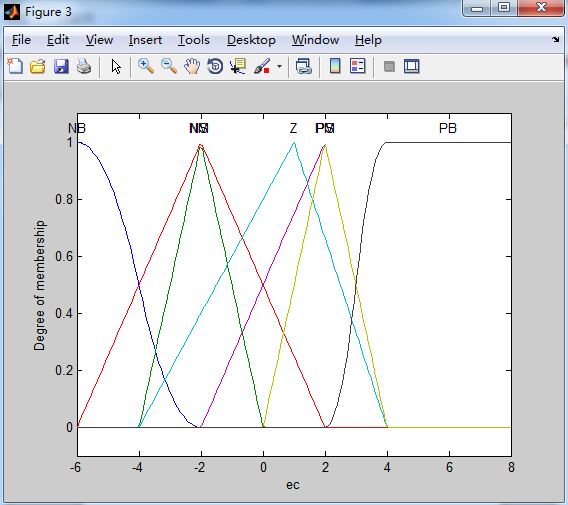
U的隶属度函数

模糊规则:
ans =
1. If (e is NB) and (ec is NM) then (u is NB) (1)
2. If (e is NB) and (ec is NM) then (u is NB) (1)
3. If (e is NB) and (ec is NS) then (u is NS) (1)
4. If (e is NB) and (ec is Z) then (u is NM) (1)
5. If (e is NB) and (ec is PS) then (u is NM) (1)
6. If (e is NB) and (ec is PM) then (u is Z) (1)
7. If (e is NB) and (ec is PB) then (u is Z) (1)
8. If (e is NM) and (ec is NM) then (u is NM) (1)
9. If (e is NM) and (ec is NM) then (u is NM) (1)
10. If (e is NM) and (ec is NS) then (u is NS) (1)
11. If (e is NM) and (ec is Z) then (u is NM) (1)
12. If (e is NM) and (ec is PS) then (u is NS) (1)
13. If (e is NM) and (ec is PM) then (u is NM) (1)
14. If (e is NM) and (ec is PB) then (u is PS) (1)
15. If (e is NS) and (ec is NM) then (u is NB) (1)
16. If (e is NS) and (ec is NM) then (u is NM) (1)
17. If (e is NS) and (ec is NS) then (u is NM) (1)
18. If (e is NS) and (ec is Z) then (u is NM) (1)
19. If (e is NS) and (ec is PS) then (u is NS) (1)
20. If (e is NS) and (ec is PM) then (u is Z) (1)
21. If (e is NS) and (ec is PB) then (u is PS) (1)
显示的矩阵和数组的内容
fuzzy Controller table:e=[-3,+3],ec=[-3,+3]
完成的模糊推理计算后的数据矩阵
Ulist =
-1.8307 -2.3306 -1.8307 -1.1663 -0.5696 -0.5362 -0.4345
-1.8289 -2.3550 -1.8289 -1.1663 -0.5691 -0.5041 -0.4345
-1.8422 -2.2175 -1.8422 -1.1839 -0.5864 -0.5656 -0.4345
-2.1515 -2.1515 -2.1515 -1.3332 -0.6721 -0.6721 -0.4345
-2.0997 -2.0997 -2.0997 -1.6006 -0.7568 -0.7568 -0.6461
-2.0614 -2.0614 -2.0614 -1.8161 -0.9071 0.0000 0.0000
-2.0400 -2.0400 -2.0400 -1.9531 -0.9737 0.0000 0.0000
三、总结
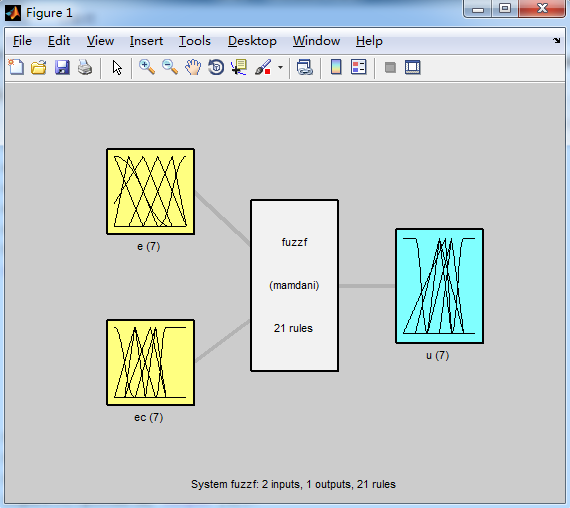
本次试验有两个输入和一个输出,输入(e,ec),输出(U)。e的取值范围是[-4,6],ec的取值范围是[-6,8],u的取值范围是[-6,6],三个都有七个模糊集,模糊规则各有3个模糊集,所以最终一共有21个模糊规则。