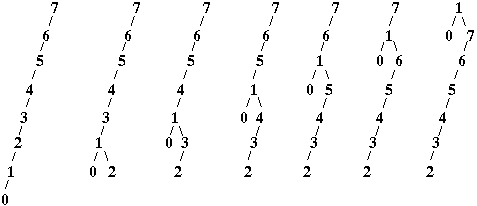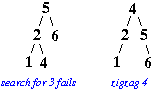【433】COMP9024 复习

01. Week01 - Lec02 - Revision and setting the scene
02. Week02 - Lec01 - Data structures - memory allocation
03. Week02 - Lec02 - Input - Output
04. Week03 - Lec01 - ADTs
05. Week03 - Lec02 - Dynamic memory allocation
06. Week04 - Lec01 - LinkedLists
07. Week06 - Lec01 - Heaps
08. Week06 - Lec02 - Priority Queues and Heap Sort
09. Week07 - Lec01 - Graphs
10. Week07 - Lec02 - Graph search
11. Week08 - Lec02 - Applications of Graph Search
12. Week09 - Lec01 - Weighted Graph Algorithms
13. Week09 - Lec02 - Binary Search Trees
14. Week10 - Lec01 - Splay Trees

01. Week01 - Lec02 - Revision and setting the scene

C语言除法只有一个符号，与 Python 不同，如果想要得到 float 类型，首先变量需要为 float，另外就是除数或者被除数之一是 float 类型
如果变量为 int，则自动获取整数部分，不管除数与被除数是否为 float
```
float a = 10.0/3, a = 3.333333
int a = 10.0/3, a = 3
```

Array 可以通过下面方式声明

int fac[20];    //对于每个元素具体赋值
int v[5] = {1, 2, 3, 4, 5};    //直接赋值的话，需要加上大括号

字符数组需要保留最后为'\0'

char s[6] = {'h', 'e', 'l', 'l', 'o'};
char t[6] = "hello";

数组作为函数参数的时候，同时需要加入数组的长度
```
int sumArray(int harry[], int length) {...}
or
int sumArray(int *harry, int length) {...}
```
其中 Harry[] 与 *harry 可以达到一样的效果

二维数组可以看成数组的数组

m[2][3] 包含2个元素，每个元素都有一个包含3个元素的数组
m[0] 包含 m[0][0], m[0][1], m[0][2]，第1行
m[1] 包含 m[1][0], m[1][1], m[1][2]，第2行
int m[2][3] = {{1, 2, 3}, {4, 5, 6}};

m[3][2][4] 包含3个元素，其中每个元素一个2*4的数组
分层，最外层3个元素，然后一层是2个元素，最里面是4个元素

int m[3][2][4] = {{{1, 2, 3, 4}, {5, 6, 7, 8}},
{{9, 10, 11, 12}, {13, 14, 15, 16}},
{{17, 18, 19, 20}, {21, 22, 23, 24}}};

struct date {...};
其中 struct date 本身是一个新的数据类型，因此定义新的变量需要这样：
struct date birthday;

#define 用来定义常量
typedef 用来将数据类型重命名
注意，前面有符号的，后面没有分号，前面没有符号的，后面需要分号

#define MAX 20
typedef struct date Date;
typedef float Real;
typedef struct {
    int day, month, year;
} Date;
Date birthdays[MAX];    // an array of structs

结构体访问

structTypeName s, *sp;
sp = &s;
s.element
(*sp).element
sp->element

int main(int argc, char *argv[])
argc: number of arguments
argv[]: allow access to arguments represented as strings
atoi(Origin_string): string to number
sscanf(Origin_string, "%d", &num): string to everything(num)

02. Week02 - Lec01 - Data structures - memory allocation

a char takes 1 byte (8 bits)
an int takes 4 bytes (32 bits)
a pointer takes 8 bytes (64 bits) (64 bit computers)
sizeof structs
组成 8 bytes，无法组成的就空缺，可以设想成 8 bytes 一个卡壳，而且按顺序放
```
struct s1 {
    char c;
    int i;
    char *s;
};
```
其中 1 + 4 (+ 3) + 8 = 16
```
struct s2 {
    char c;
    char *s;
    int i;
};
```
其中 1 (+ 7) + 8 + 4 (+4) = 24

03. Week02 - Lec02 - Input - Output

3 main sources of input for programs:
from the command line: argc, argv[]
from standard input (also called stdin)
stdin can be the keyboard, a data file, or the output of other program
from an 'internally-defined' file
open a file, use fscanf(), and don't forget to close the file
command line
sscanf(argv[1], "%d", &num) != 1
如果赋值成功的话，会返回赋值的个数，将字符串 argv[1] 转换为数字 num
standard input (stdin)
```
scanf("%d", &num) != 1
or
scanf("%d%d, &num1, &num2) != 2    //表示两个数字，中间可以间隔whitespace
```
没有 string 参数，直接获取 stdin 的信息，或者是输入的，或者是文件
pipe command: |, 将前一个输出当做后一个输入

使用 getchar()
一次获取一个字符，检测是否到达 '\n'
```
char c = getchar();
while (c != '\n') {...}
```
a user file
fopen(), fscanf(), fclose()
stdout, stderr
fprinf(stream, ...)
stdin: scanf()
stdout: printf()
scanf(): to read from stdin，只能读取 keyboard 或者文件，与 printf 对应
多个含有空格的字符，无线调用 scanf 可以读取数字
sscanf(): to read from the command line，从字符串获取信息
printf(): to write to stdout，只能输出到控制台或者文件
fprintf(): to write to stderr

04. Week03 - Lec01 - ADTs

实现 struct
```
typedef struct point {
    float x;
    float y;
} *Point;
```
struct point 用来计算 malloc 中空间大小
Point 以指针形式，可以直接修改地址对应的数据
```
Point p;
p = malloc(sizeof(struct point));
p->x
p->y
```
Stacks and queues
stacks: Last in, first out
    通过数组实现，快速简单，不过要固定长度
    通过 linkedlist 实现，慢，不过可以灵活增加内存
    a postfix calculator
queues: First in, first out
    ‘circular’ queue
数组实现：
    stacks：尾部push，尾部pop
    queues：头部qush，尾部pop

05. Week03 - Lec02 - Dynamic memory allocation

声明的变量放在 stack 里面
malloc 的内存空间放在 heap 里面
For every malloc() there should be a corresponding free()
when free'd, that heap space may be re-used by the system
free(p);
p = NULL;
When a program teminates, all memory the program uses is automatically freed.
It is not dangerous to forget to free memory at the end of a program,
but it's poor style. Sometimes it's dangerous.
Realloc
If the space returned by a malloc() is too little, you can create more by calling realloc().
```
char *p = malloc(SIZEINBYTES)
.
.
char *pext = realloc(p, BIGGERSIZEINBYTES);
.
.
```

06. Week04 - Lec01 - LinkedLists

与数组相比的优点：
- 动态分配空间，可以是任意大小
- 数组需要连续的空间，不好把控
- 更容易删除节点
与数组相比的缺点：声明：
- 不能随机访问，数组可以
- 只能连续访问，但是数组更快
- 操作量更大
- 每个元素都有一个额外的指针

Sedgwick

  typedef struct node *Link; 
  struct node{ 
      int data; 
      Link next;
  }

Albert

  typedef struct node {
      int data;
      struct node *next;
  } List;

通过 LinkedList 来实现 Quack
- push：将 node 放在 head 之后，就是最前面
- qush：需要找到最后一个节点，然后直接连接上
- pop：Quack qs，qs 不能是 NULL，不能使 Empty, 删除第一个 node 并且释放
- destroyQuack：释放出 head 之外的所有节点
- makeEmptyQuack：pop 掉所有节点
- isEmptyQuack：是否头节点指向 NULL
- showQuack：按顺序输出 data
Head nodeA linkedlist ADT interface
- 创建出来的
- 存储的是 dummy data，最大值，没意义
- 不能被删除，不是 linkedlist 信息内容
- 如果 quack 为空，指向 NULL
- 如果 quack 不为空，指向 top node
- 返回 head node 给 client
typedef struct node *List;
...
因此 interface 里面可以用到 List
A linkedlist ADT
```
struct node {
    int data;
    struct node *Next;
};
...
```
因此 ADT 里面可以用访问结构体内部信息

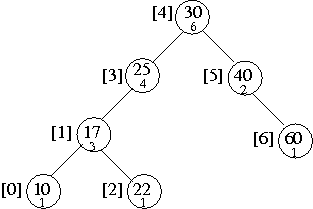
07. Week06 - Lec01 - Heaps

heap memory
- a programmer can request heap memory by using malloc()
- the memory is 'returned' to the heap by using free()
heap data structure
- higher-level than array and linked list
  - a heap data structrue is built on an array, or linked list
- two-dimensional because it forms a tree of data
  - a binary tree (at most 2 children per node)
Max-heaps
- the element with the largest key is at the root
- the children of a node have keys smaller than the parent
Min-heaps
- the element with the smallest key is at the root
- the children of a node have keys larger than the parent
2 properties
- HOP (Heap-Order Property): 子节点的值比父节点小或者大
- CTP (Complete-Tree Property): 完全二叉树，可以保证子节点与父节点的位置是2倍关系
- heaps 用来实现 priority queues，使用在最短路径算法
Heap Implementation
- 根节点索引值为 1
- 给定节点索引 i：
  - 它的左节点是 2i
  - 它的右节点是 2i + 1
  - 它的父节点是 i/2（使用整数结果）
Operation on heaps
- Get the maximum element
  - 根节点，位置 1
- Insert an element
  - 把新要素放到最下面最右边的位置，放在数组的最后
  - fixUp：随着单一路径向根部传递，按照 Heap-Order Property
- Delete the maximum element
  - delMax 大根堆
  - delMin 小根堆
  - 删除叶子节点，可以直接操作
  - 删除根节点
    - 删除root，将最后的元素替换掉，并将其删除，然后
    - fixDown from the root （Heap-Order Property）
    - return the saved root node
  - fixDown：随着单一路径从根部向叶子节点传递

08. Week06 - Lec02 - Priority Queues and Heap Sort

A priority queue supports 2 abstract operations:
- insert: a new item (push, qush)
- delMax (pop)
A Priority Queue ADT
- insertPQ：通过 fixUp，构建大根堆
- delMaxPQ：删掉第一个值，然后执行 fixDown
Three implementations of the PQ ADT
- an unordered array
  - ```
  struct pqRep { 
     int nItems;  // actual count of Items 
     int *items;  // array of Items 
     int maxsize; // maximum size of array 
  };
```
- 插入数据，放置在最后
- 删除最大值，通过遍历来获取
- an ordered array
  - 插入数据，查找数据按照顺序的位置，然后将后面的数据逐个后移，将数据插入
  - 删除最大值，就是最后一个元素
- a heapified array
  - 插入数据，调用 fixUp，将数据进行排序
  - 删除最大值，删除一个元素，最后一个元素补入，通过 fixDown 操作

09. Week07 - Lec01 - Graphs

Complete graph
- 任何两点都有相连的边
- |E| = V(V-1)/2 （排列组合里面 V 个顶点，里面随机选取 2 个的可能性）
Clique
- a complete subgraph
- a subset of vertices that form a complete graph
Sparseness/denseness of a gragh
- dense graphs: |E| is closer to V(V-1)/2
- sparse graphs: |E| is closer to V (minimum is V-1)
Tree
- a connected (sub)graph with no cycles
Spanning Tree
- a tree that contains all vertices in the graph （可以是所有孤立的点，只删除 edges，点集不变）
- 包含所有 graph 中顶点的 tree，例如最小生成树
Terminology
- graph；顶点集与边集
- subgraph：顶点集与边集都是上面graph 的子集
- induced subgraph：导出子图，顶点集是子集（只删除顶点的子集，相连的边会自动删除）
  ref: https://www.youtube.com/watch?v=dPHkyRvLtIU
- cycle：首尾相接
- connected graph：连接所有顶点的路径
- adjacency：两个相连接的顶点
- degree：一个顶点与其相连的顶点个数
Hamiltonian path and cycle
- 每个顶点只访问一次，path
- 如果首尾相接，cycle（所有顶点的degree >= n/2）
Eulerian path and cycle
总结：
- 遍历每条边正好一次，path（恰好只有2个顶点有奇数的degree）
- 如果收尾相接，cycle（所有的顶点都是偶数的degree）
- 两者都不是：看奇数degree顶点个数，如果1个或者大于2个，都是没有的
1. 不可能同时有欧拉path或者cycle
2. 有Hamiltonian cycle一定有path
3. 判断H 回路的方法就是所有顶点的度都≥n/2
4. 判断欧拉path：只有两个是奇数的度
5. 判断欧拉cycle：所有都为偶数的度
其他
- Undirected Graphs
  - edge(u, v) = edge(v, u)
  - no self-loops (i.e. no edge(v, v))
- Directed Graphs
  - edge(u, v) ≠ edge(v, u)
  - can have self-loops (i.e. edge(v, v))
- Weighted Graphs
  - 有权值的
  - road map
- Multi-graph
  - 两个顶点间可以拥有多条边相连
  - 去一个地方的不同方式，线路等
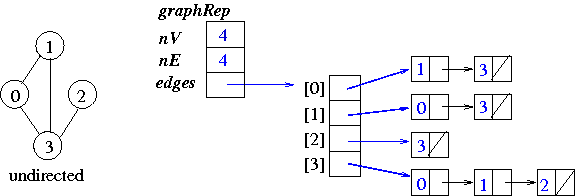
Implementing Graphs
- Vertex that is represented by an int
- Edge that is represented by 2 vertices
- Graph that is represented by an Adjacency matrix, or as an Adjacency list.
-     building:
          create a graph
          create an edge
          add an edge to a graph
      deleting
          remove an edge from a graph
          remove and free a graph
      printing
          'show' a graph
- Edge
```
typedef int Vertex;               // define a VERTEX

typedef struct {                  // define an EDGE
  Vertex v;
  Vertex w;
} Edge;
```

GraphAM.c: based on an Adjacency Matrix

struct graphRep { 
    int nV;       // #vertices 
    int nE;       // #edges 
    int **edges;  // matrix of Booleans ... THIS IS THE ADJACENCY MATRIX
};

GraphAL.c: based on an Adjacency Linked List

typedef struct node *List;
struct node { 
  Vertex name; 
  List next; 
};

struct graphRep { 
  int nV;    // #vertices
  int nE;    // #edges
  List *edges; // array of linked lists ... THIS IS THE ADJACENCY LIST
};

insertEdge: 两个顶点都要加，并且是插入到头部的位置

Reading a graph

scanf("%d", &v) != EOF，说明没有读取到文件结尾
scanf("%d", &v) != '\n'，说明没有读取到换行符
scanf 会自动跳过空格、制表符或者回车

int readNumV(void) { // returns the number of vertices numV or -1
   int numV;
   char w[WHITESPACE];
   scanf("%[ \t\n]s", w);  // skip leading whitespace
   if ((getchar() != '#') ||
       (scanf("%d", &numV) != 1)) {
       fprintf(stderr, "missing number (of vertices)\n");
       return -1;
   }
   return numV;
}

int readGraph(int numV, Graph g) { // reads number-number pairs until EOF
   int success = true;             // returns true if no error
   int v1, v2;
   while (scanf("%d %d", &v1, &v2) != EOF && success) {
       if (v1 < 0 || v1 >= numV || v2 < 0 || v2 >= numV) {
          fprintf(stderr, "unable to read edge\n");
          success = false;
       }
       else {
          insertEdge(newEdge(v1, v2), g);
       }
   }
   return success;
}

10. Week07 - Lec02 - Graph search

Depth-first search (BFS)

stack

//HANDLES CONNECTED GRAPHS ONLY
void dfsQuack(Graph g, Vertex v, int numV) {
   int *visited = mallocArray(numV);
   Quack s = createQuack();
   push(v, s);
   showQuack(s);
   int order = 0;
   while (!isEmptyQuack(s)) {
      v = pop(s);
      if (visited[v] == UNVISITED) {   // we visit only unvisited vertices
         printArray("Visited: ", visited, numV);
         visited[v] = order++;
         for (Vertex w = numV - 1; w >= 0; w--) { //push adjacent vertices
            if (isEdge(newEdge(v,w), g)) {        // ... in reverse order
               push (w, s);                       // ... onto the stack
            }
         }
      }
      showQuack(s);
   }
   printArray("Visited: ", visited, numV);
   free(visited);
   return;
}

dfsQuack() (disconnected graphs)

设置一个参数 allVis，判断是否全部顶点都访问了

//HANDLES CONNECTED GRAPHS ONLY
void dfsQuack(Graph g, Vertex v, int numV) {
   int *visited = mallocArray(numV);
   Quack s = createQuack();
   push(v, s);
   showQuack(s);
   int order = 0;
   while (!isEmptyQuack(s)) {
      v = pop(s);
      if (visited[v] == UNVISITED) {   // we visit only unvisited vertices
         printArray("Visited: ", visited, numV);
         visited[v] = order++;
         for (Vertex w = numV - 1; w >= 0; w--) { //push adjacent vertices
            if (isEdge(newEdge(v,w), g)) {        // ... in reverse order
               push (w, s);                       // ... onto the stack
            }
         }
      }
      showQuack(s);
   }
   printArray("Visited: ", visited, numV);
   free(visited);
   return;
}

Recursive Depth-First Search

包含了 disconnected graphs 的情况，另外函数内部加入指针，可以改变地址同时相当于全局变量

void dfs(Graph g, Vertex rootv, int numV) {//'wrapper' for recursive dfs
   int *visited = mallocArray(numV);  // ... handles disconnected graphs
   int order = 0;
   Vertex startv = rootv;             // this is the starting vertex
   int allVis = 0;                    // assume not all visited
   while (!allVis) {                  // as long as there are vertices
      dfsR(g, startv, numV, &order, visited);
      allVis = 1;                     // are all visited now?
      for (Vertex w = 0; w < numV && allVis; w++) { // look for more
         if (visited[w] == UNVISITED) { 
            printf("Graph is disconnected\n"); // debug
            allVis = 0;               // found an unvisited vertex
            startv = w;              // next loop dfsR this vertex
         }
      }
   }
   printArray("Visited: ", visited, numV);
   free(visited);
   return;
}

void dfsR(Graph g, Vertex v, int numV, int *order, int *visited) {
   visited[v] = *order;                // records the order of visit
   *order = *order+1;
   for (Vertex w = 0; w < numV; w++) {
      if (isEdge(newEdge(v,w), g) && visited[w]==UNVISITED) {
         dfsR(g, w, numV, order, visited);
      }
   }
   return;
}

Breadth First Search

queue

void bfsQuack(Graph g, Vertex v, int numV) { //name change
   int *visited = mallocArray(numV);
   Quack q = createQuack();
   qush(v, q);                             //qush, not push
   showQuack(q);
   int order = 0;
   while (!isEmptyQuack(q)) {
      v = pop(q);
      if (visited[v] == UNVISITED) {
         printf("Visit %d\n", v);  // debug
         visited[v] = order++;
         for (Vertex w = 0; w < numV; w++) {//vertex order
            if (isEdge(newEdge(v,w), g)) {
               qush(w, q);                  //qush, not push
            }
         }
      }
      showQuack(q);
   }
   printArray("Visited: ", visited, numV);
   free(visited);
   makeEmptyQuack(q);
   return;
}

11. Week08 - Lec02 - Applications of Graph Search

Breadth-First Search: Shortest path

ref: https://www.cnblogs.com/alex-bn-lee/p/11242024.html#A42

void shortestPath(Graph g, Vertex start, Vertex goal, int numV) {
   int *visited = mallocArray(numV);
   int *parent = mallocArray(numV);          // THIS IS NEW

   Quack q = createQuack();
   qush(start, q);
   int order = 0;
   visited[start] = order++;
   int found = 0;
   while (!isEmptyQuack(q) && !found) {      // FOUND TELLS US TO STOP
      Vertex v = pop(q);
      for (Vertex w = 0; w < numV && !found; w++) {
         if (isEdge(newEdge(v,w), g)) {      // for adjacent vertex w ...
            if (visited[w] == UNVISITED) {   // ... if w is unvisited ...
               qush(w, q);                   // ... queue w
               printf("Doing edge %d-%d\n", v, w); // DEBUG
               visited[w] = order++;         // w is now visited
               parent[w] = v;                // w's PARENT is v
               if (w == goal) {              // if w is the goal ...
                  found = 1;                 // ..FOUND IT! NOW GET OUT
               }
            }
         }
      }
   }
   if (found) {
      printf("SHORTEST path from %d to %d is ", start, goal);
      printPath(parent, numV, goal); // print path from START TO GOAL
      putchar('\n');
   }
   else {
      printf("no path found\n");
   }
   printArray("Visited: ", visited, numV);   // debug
   printArray("Parent : ", parent, numV);    // debug
   free(visited);
   free(parent);
   makeEmptyQuack(q);
   return;
}

// head recursion
void printPath(int parent[], int numV, Vertex v) {
   if (parent[v] != UNVISITED) { // parent of start is UNVISITED
      if (0 <= v && v < numV) {
         printPath(parent, numV, parent[v]);
         printf("-->");
      }
      else {
         fprintf(stderr, "\nprintPath: invalid goal %d\n", v);
      }
   }
   printf("%d", v); // the last call will print here first
   return;
}

void printPath(int parent[], int numV, Vertex v) {
       printf("%d", v);
       if (0<=v && v<numV) {
          Vertex p = parent[v];
          while (p != UNVISITED) {
              printf("<--%d", p);
              p = parent[p];
          }
       }
       else {
          fprintf(stderr, "printPath: illegal vertex in parent[]\n");
       }
    }

Depth-First Search: Cycle detection

ref: https://www.cnblogs.com/alex-bn-lee/p/11242024.html#A43

// a wrapper for the recursive call to hasCycle()
void searchForCycle(Graph g, int v, int numV) {
   int *visited = mallocArray(numV);
   int order = 0;

   if (hasCycle(g, numV, v, v, &order, visited)) {
      printf("found a cycle\n");
   }
   else {
      printf("no cycle found\n");
   }
   printArray("Visited ", visited, numV);
   free(visited);
   return;
}

int hasCycle(Graph g, int numV, Vertex fromv, Vertex v, int *order, int *visited) {
   int retval = 0;
   visited[v] = *order;
   *order = *order+1;
   for (Vertex w = 0; w < numV && !retval; w++) {
      if (isEdge(newEdge(v,w), g)) {
         if (visited[w] == UNVISITED) {
            printf("traverse edge %d-%d\n", v, w);
            retval = hasCycle(g, numV, v, w, order, visited);
         }
         else {
            if (w != fromv) { // exclude the vertex we've just come from
               // WE HAVE REVISITED A VERTEX ==> CYCLE

               printf("traverse edge %d-%d\n", v, w);
               retval = 1;
            }
         }
      }
   }
   return retval;
}

Depth-First Search: Eulerian cycles

https://www.cnblogs.com/alex-bn-lee/p/11242024.html#A44

void findEulerianCycle(Graph g, int numV, Vertex startv) {
   Quack s = createQuack();
   printf("Eulerian cycle: ");

   push(startv, s);
   while (!isEmptyQuack(s)) {
      Vertex v = pop(s); // pop and then ...
      push(v, s);        // ... push back on, so no change
      Vertex w = getAdjacent(g, numV, v); // get largest adj. v
      if (w >= 0) {      // if true, there is an adj. vertex
         push(w, s);     // push this vertex onto stack
         removeEdge(newEdge(v, w), g); // remove edge to vertex
      }
      else {             // top v on stack has no adj. vertices
         w = pop(s);
         printf("%d ", w);
      }
   }
   putchar('\n');
}

Vertex getAdjacent(Graph g, int numV, Vertex v) {
   Vertex retv = -1; // assume no adj. vertices
   for (Vertex w = numV-1; w >= 0 && retv == -1; w--) { 
      if (isEdge(newEdge(v, w), g)) {
         retv = w;   // found largest adj. vertex
      }
   }
   return retv;
}

Depth-First Search: Finding a path

https://www.cnblogs.com/alex-bn-lee/p/11242024.html#A45

void dfsR(Graph g, Vertex v, int numV, int *order, int *visited) {
   visited[v] = *order;
   *order = *order+1;
   for (Vertex w = 0; w < numV; w++) {
      if (isEdge(newEdge(v,w), g) && visited[w]==UNVISITED) {
         dfsR(g, w, numV, order, visited);
      }
   }
   return;
}

int isPath(Graph g, Vertex v, Vertex goalv, int numV, int *order, int *visited) {
   int found = 0;
   visited[v] = *order;
   *order = *order+1;
   if (v == goalv) {
      found = 1;
   }
   else {
      for (Vertex w = 0; w < numV && !found; w++) {
         if (isEdge(newEdge(v,w), g)) {
            if (visited[w] == UNVISITED) {
               found = isPath(g, w, goalv, numV, order, visited);
               printf("path %d-%d\n", w, v);
            }
         }
      }
   }
   return found;
}

12. Week09 - Lec01 - Weighted Graph Algorithms

Dijkstra 算法
- 【428】Dijkstra 算法
Prim 算法Kruskal 算法
- 【431】Prim 算法 & Kruskal 算法
Firefox 崩溃了，数据丢了，坑爹~

13. Week09 - Lec02 - Binary Search Trees

Height：具体根节点的高度，root 为 0
Node level or depth：跟 Height 差不多
Types of trees
- ordered tree: 有序树
- binary tree: 二叉树
- full binary tree: 满二叉树，每个节点都有2个孩子，可能参差不齐
- perfect binary tree: 完美二叉树，类似三角形那种，都铺满了
- ordered binary tree:有序二叉树，也叫做 binary search tree，二叉搜索树
  - left subtree values <= parent value
  - right subtree values >= parent value
- full m-ary tree: 类似满二叉树
Binary Trees
- balanced：尽量满，最短的
- degenerate：退化的，一边倒，最长的
Height of a binary tree
- 最长路径的长度
- degenerate: height is n-1
- balanced: height is ln(n)

Data structure for a binary tree

typedef struct node *Tree;
   struct node {
      int data;
      Tree left;
      Tree right;
   };

Comparing Binary Search Trees and Heaps

Searching in BSTs

结束条件就是顶点为 NULL，返回 0，或者查到了 v 值，返回 1

int searchTree(Tree t, int v){ // Search for the node with value v
   int ret = 0;
   if (t != NULL) {
      if (v < t->data) {
         ret = searchTree(t->left, v);
      }
      else if (v > t->data) {
         ret = searchTree(t->right, v);
      }
      else { // v == t->data
         ret = 1;
      }
   }
   return ret; 
} // returns non-zero if found, zero otherwise

Creating a node in a BST

构建结构体，建立数，结构体是一个 node 点，但是树是一个指针 Tree，连接着所有的点
创建树，首先就是分配内存空间，然后就是赋值
默认左右节点都为 NULL

typedef struct node *Tree;
struct node {
   int data;
   Tree left;
   Tree right;
};

Tree createTree(int v) {
   Tree t;
   t = malloc(sizeof(struct node));
   if (t == NULL) {
      fprintf(stderr, "Out of memory\n");
      exit(1);
   }
   t->data = v;
   t->left = NULL;
   t->right = NULL;
   return t;
}

Freeing a node in a BST

结束条件就是遇到叶子节点，因为其左右节点均为 NULL

void freeTree(Tree t) { // free in postfix fashion
      if (t != NULL) {
         freeTree(t->left);
         freeTree(t->right);
         free(t);
      }
      return;
   }

Inserting a node in a BST

重点是返回值是 Tree
结束条件就是遇到叶子节点，因为其左右节点均为 NULL，因此会创建新的 node 点，然后将值依次返回
由于返回值为 Tree，因此自动连接的起来

Tree insertTree(Tree t, int v) {
   if (t == NULL) {
      t = createTree(v);
   }
   else {
      if (v < t->data) {
        t->left = insertTree (t->left, v);
      }
      else {
        t->right = insertTree (t->right, v);
      }
   }
   return t;
}

do it iteratively
通过遍历找到对应的叶子节点，然后连接上 node

Tree insertTreeI(Tree t, int v) { // An iterative version of the above
   if (t == NULL) {
      t = createTree(v);
   }
   else { // t != NULL
      Tree parent = NULL;  // remember the parent to link in new child
      Tree step = t;
      while (step != NULL) { // this is the iteration
         parent = step;
         if (v < step->data) {
           step = step->left;
         }
         else {
           step = step->right;
         }
      } // step == NULL
      if (v < parent->data) {
         parent->left = createTree(v);
      }
      else {
         parent->right = createTree(v);
      }
   }
   return t;
}

Printing the tree

遍历打印，先左再中后右

void printTree(Tree t) { // not the final version
    if (t != NULL) {
        printTree (t->left);
        printf ("%d  ", t->data);
        printTree (t->right);
    }
    return;
}

2D printing
每次递归，depth 自增，打印数据的时候，先将制表符打印出来，出现层次感

void printTree(Tree t, int depth) { // extra depth parameter
    if (t != NULL) {
        depth++;
        printTree (t->left, depth);
        for (int i=1; i<depth; i++){ // 'depth'*whitespace
           putchar('\t');
        }
        printf ("%d\n", t->data); // node to print
        printTree (t->right, depth);
    }
    return;
}

反方向打印

void printTree2(Tree t, int depth) {
    if (t != NULL) {
        depth++;
        printTree2 (t->right, depth);
        int i;
        for (i=1; i<depth; i++){
            putchar('\t');
        }
        printf ("%d\n", t->data);
        printTree2 (t->left, depth);
    }
    return;
}

效果如下：

1
					2
				3
			4
		5
	6
7
	8
====================================
	8
7
	6
		5
			4
				3
					2
						1

Count the number of nodes in a BST
- 遍历相加，每个节点为 1
  结束条件就是叶子节点，因为其左右节点均为 NULL，自动返回 1，然后不停回溯。。
- ```
int count(Tree t){
   int countree = 0;
   if (t != NULL) {
      countree = 1 + count(t->left) + count(t->right);
   }
   return countree;
}
```

Find the height of a BST

对于叶子节点本身，高度为 0，因此 NULL 节点的高度就为 -1
parent 节点的高度 = 1 + MAX (left height, right height)

int max(int a, int b){
      if (a >= b) {
         return a;
      }
      return b;
   }

   int height(Tree t){
      int heightree = -1;
      if (t != NULL){
         heightree = 1 + max(height(t->left), height(t->right));
      }
      return heightree;
   }

How balanced is a BST?
How do the number of nodes in the left sub-tree and the right sub-tree compare?

分别计算出左右子树的节点数，然后相减即可

int balance (Tree t){ // calculates the difference between left and right
      int diff = 0;

      if (t != NULL) {
         diff = count(t->left) - count(t->right); // count declared elsewhere
         if (diff < 0) {
            diff = –diff;
         }
      }
      return diff;
   }

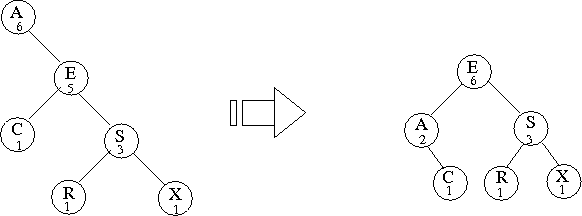
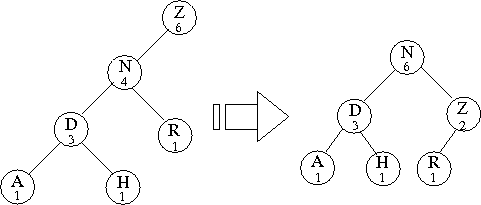
Deleting a node from a BST

3 cases
- node is a leaf: unlink node from parent
- node has 1 child: replace the node by its child
- node has 2 children: rearrange the tree in some way
  - DLMD: Deepest Left-Most Descendent
  - DRMD: Deepest Right-Most Descendent
把 DLMD 摘下来，左边连接 t1，右边连接 t2
DLMD 为最左，因此其次需要将其 parent 节点的 left 与 DLMD 的 right 相连
p->left = nuroot->right
注意需要保留 DLMD 的 parent 节点

Tree deleteTree(Tree t, int i){ // delete node with value 'v'
  if (t != NULL) {
    if (v < t->data) {
      t->left = deleteTree(t->left, v);
    }
    else if (v > t->data) {
      t->right = deleteTree(t->right, v);
    }
    else {    // v == t->data, so the node 't' must be deleted
      // next fragment of code violates style, just to make logic clear
      Tree n;                                             // temporary
      if (t->left==NULL && t->right==NULL) n=NULL;        // 0 children
      else if (t->left ==NULL)             n=t->right;    // 1 child
      else if (t->right==NULL)             n=t->left;     // 1 child
      else                                 n=joinDLMD(t->left,t->right);
      free(t);
      t = n;
   }
 }
 return t;
}

// Joins t1 and t2 with the deepest left-most descendent of t2 as new root.
Tree joinDLMD(Tree t1, Tree t2){
   Tree nuroot;
   if (t1 == NULL) {         // actually should never happen
      nuroot = t2;
   }
   else if (t2 == NULL) {    // actually should never happen
      nuroot = t1;
   }
   else {                    // find the DLMD of the right subtree t2
      Tree p = NULL;
      nuroot = t2;
      while (nuroot->left != NULL) {
          p = nuroot;
          nuroot = nuroot->left;
      }                      // nuroot is the DLMD, p is its parent
      if (p != NULL){
          p->left = nuroot->right; // give nuroot's only child to p
          nuroot->right = t2;      // nuroot replaces deleted node
      }
      nuroot->left = t1;           // nuroot replaces deleted node
   }
   return nuroot;
}

14. Week10 - Lec01 - Splay Trees

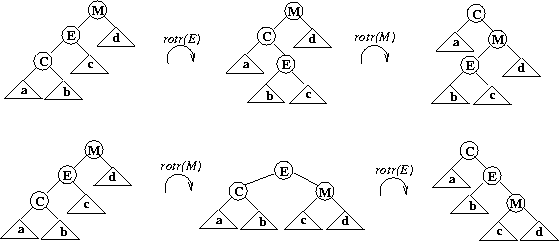
Right rotation
- its right child moves to the root's left child the root moves to its right child
- 折下来，parent变成child

Left rotation

its left child moves to the root's right child the root moves to its left child
翻上去，child变成parent
相当于告诉了root，然后获取转折点，第一个是 E 是root，然后获取 C，同理。。
然后就是连接起来即可

// NOT REAL CODE: CHECKS REQUIRED: ACTUAL CODE BELOW
Tree rotateR(Tree root) {    // old root
   Tree newr = root->left;   // newr is the new root
   root->left = newr->right; // old root has new root's right child
   newr->right = root;       // new root has old root as right child
   return newr;              // return the new root
}
// NOT REAL CODE: CHECKS REQUIRED: ACTUAL CODE BELOW
Tree rotateL(Tree root) {    // old root
   Tree newr = root->right;  // newr will become the new root
   root->right = newr->left; // old root has new root's left child
   newr->left = root;        // new root has old root as left child
   return newr;              // return the new root
}

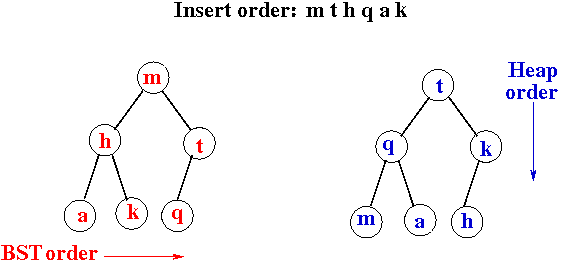
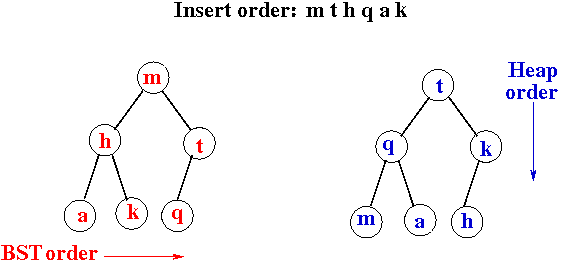
root insertion
- assume that we have just inserted node G in the BST below
  - rotate right the subtree with root the parent of G (i.e. H)
  - rotate right the subtree with root the parent of G (i.e. R)
  - rotate left the subtree with root the parent of G (i.e. E)
- 一次只是转一次，保证 G 不停向上走

Counting the number of nodes in a subtree

typedef struct node *Tree;
struct node {
   int data;
   Tree left;
   Tree right;
   int count;
};

Tree createTree(int v) {
   Tree t = malloc (sizeof(struct node));
   if (t == NULL) {
      fprintf(stderr, "Out of memory\n");
      exit(1);
   }
   t->data = v;
   t->left = NULL;
   t->right = NULL;
   t->count = 1;
   return t;
}

int sizeTree(Tree t) {
   int retval = 0;
   if (t != NULL){
      retval = t->count;
   }
   return retval;
}

  Tree insertTree(Tree t, int v) {
     if (t == NULL) {
        t = createTree(v);
     }
     else {
        if (v < t->data) {
           t->left = insertTree (t->left, v);
        }
        else {
           t->right = insertTree (t->right, v);
        }
        t->count++;    // update the counter at each ancestor
     }
     return t;
  }

Selecting the i-th element from a BST
获取某一索引的节点值
类似数组里面 arr[3] 的意思
Balanced Trees

check the number of nodes in the left subtree
- if the left subtree contains numOnLeft elements, and
  - numOnLeft>i, we recursively look in this left subtree for element i, else if
  - numOnLeft<i, we recursively look in the right subtree for element i-(numOnLeft+1), else if
  - numOnLeft=i, return with the current element's data
如果在左子树直接递归
如果在右子树，需要减掉所有左子树+root的节点数

// For tree with n nodes – indexes are 0..n-1
int selectTree(Tree t, int i) { // for tree with n nodes - indexed from 0..n-1
   int retval = 0;
   if (t != NULL) {
      int numOnLeft = 0;             // this is if there is no left branch
      if (t->left != NULL) {
         numOnLeft = t->left->count; // this is if there is a left branch
      }
      if (numOnLeft > i) {           // left subtree or ...
         retval = selectTree(t->left, i);
      }
      else if (numOnLeft < i) {      // ... right subtree ?
         retval = selectTree(t->right, i-(numOnLeft+1));
      }
      else {
         retval = t->data;          // value index i == numOnLeft
      }
   }
   else {
      printf("Index does not exist\n");
      retval = 0;
   }
   return retval;
}

Approach 1: Global rebalancing

Rotating with a count

Example of left rotation

算法与上面一致，只是增加了 count 的计算
对于 E 的count就是等于前面 A 的count
对于 A 的count就直接计算他的左右节点

Tree rotateLeft(Tree t) { // Rotate left code: includes count field
   Tree retval = NULL;
   if (t != NULL) {
      Tree nuroot = t->right;    // left rotate: hence right root-child will become new root
      if (nuroot == NULL) {
         retval = t;
      }
      else {
         t->right = nuroot->left;  // the left child of nuroot becomes old root's right child
         nuroot->left = t;         // nuroot's left child is the old root
         nuroot->count = t->count; // nuroot and old root have the same count
         t->count = 1 + sizeTree(t->left) + sizeTree(t->right); // recompute count in old root
         retval = nuroot;          // return with the new root
      }
   }
   return retval;
}

Example of right rotation

Tree rotateRight(Tree t) { // Rotate right code: includes count field
   Tree retval = NULL;
   if (t != NULL) {
      Tree nuroot = t->left;
      if (nuroot == NULL) {
         retval = t;
      }
      else {
         t->left = nuroot->right;
         nuroot->right = t;
         nuroot->count = t->count;
         t->count = 1 + sizeTree(t->left) + sizeTree(t->right);
         retval = nuroot;
      }
   }
   return retval;
}

Partitioning，分隔，分区

We can rewrite select() to partition around the i^th element in the BST:
- we descend recursively
  - to the i^th element of each subtree
- rotate-with-count that element in the opposite direction to its position
  - if it is a left child: do a right rotation
  - if it is a right child: do a left rotation
- this will make the i^th element the root of the BST
将第 i 个元素作为 root
不停的左右移动

Tree partition(Tree t, int i) { // make node at index i the root
   Tree retval = NULL;
   if (t != NULL) {
      int numOnLeft = 0;
      if (t->left != NULL) {
         numOnLeft = t->left->count;
      }
      if (numOnLeft > i) {
         t->left = partition(t->left, i);
         t = rotateRight(t);
      }
      if (numOnLeft < i) {
         t->right = partition(t->right, i-(numOnLeft+1));
         t = rotateLeft(t);
      }
      retval = t;
   }
   else {
      printf("Index does not exist\n");
      retval = NULL;
   }
   return retval;
}

Balancing the BST
Approach 2: Local Rebalancing

Move the median node to the root by partitioning on i = count/2
- balance the left sub-tree
- balance the right sub-tree
先把 median 移动到 root
递归移动左右，最终所有的都是 median 在中间

Tree balance(Tree t) {
   Tree retval = NULL;
   if (t != NULL) {
      if (t->count <= 1){
         retval = t;
      }
      else {
         t = partition(t, t->count/2);
         t->left = balance(t->left);
         t->right = balance(t->right);
         retval = t;
      }
   }
   return retval;
}

Splaying an item to the top means to move an item to the root of the BST, using one of the operations:
1. zigzag
2. zigzig
zigzag operation （先下后上）
- the item is the right child of the parent, which is a left child of the grandparent
  - do a rotate-left of the parent followed by a rotate-right of the grandparent
  - /
    \ 先是左转parent
    /
    / 然后再右转grandparent /\
- the item is the left child of the parent, which is a right child of the grandparent
  - do a rotate-right of the parent followed by a rotate-left of the grandparent
  - \
    / 先是右转parent
    \
    \ 然后再左转grandparent /\
zigzig operation （先上后下）
- the item is the left child of the parent, which is a left child of the grandparent
  - do a rotate-right of the grandparent followed by a rotate-right of the parent
  - (not a rotate-right of the parent followed by a rotate-right of the grandparent
  - /
    / 先是右转grandparent /\ 然后再右转parent
    \
    \
- the item is the right child of the parent, which is a right child of the grandparent
  - do a rotate-left of the grandparent followed by a rotate-left of the parent
  - (not a rotate-left of the parent followed by a rotate-left of the grandparent
  - \
    \ 先是左转grandparent /\ 然后再左转parent
    /
    /
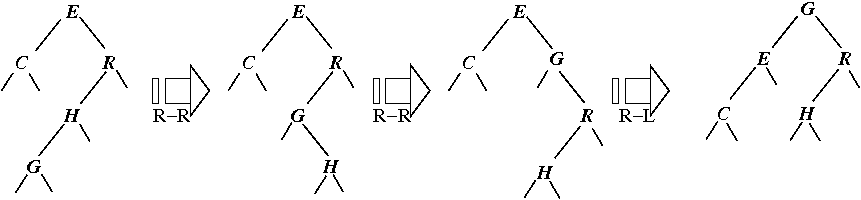
Example
- H is the right child of the left child so requires a zigzag splay
  - this is simply a left-rotate followed by a right-rotate
- P is the left child of the right child so requires a zigzag splay
  - this is simply a right-rotate followed by a left-rotate
- C is the left child of the left child so requires a zigzig splay
  - a zigzig splay does a right-rotate of the grandparent M, followed by another right-rotate of the parent E
- T is the right child of the right child so requires a zigzig splay
  - a zigzig splay does a left-rotate of the grandparent M, followed by a left-rotate of the parent Q
- The first double rotation below is not a zigzig rotation, the second is:
  区别在于，第一个图是先下后上
  而 zigzig 是先上后下
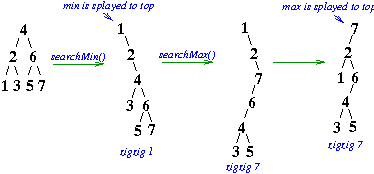
Operation splay-search
- If we search for an item, and move the item to the root by using normal left and right rotations then there is no rebalancing
  - for example: a normal search for item 1 would result in
    每次移动一个节点，就是 1 不停向上移动
- If we splay-search for an item, once found we
  - splay the item to the root
    1作为目标节点，整体是一个左左型，从grandparent右转，然后parent右转
- If the item is not found
  - the last item (leaf) on the path is splayed to the root
- For example, in the following splay tree we search for item 3, and because it fails, the 'last' item accessed, which is 4, is splayed:
  找3，结果找到了4，那就移动4，就是判断点是否为叶子节点，是的话就动
Operation splay-insertion
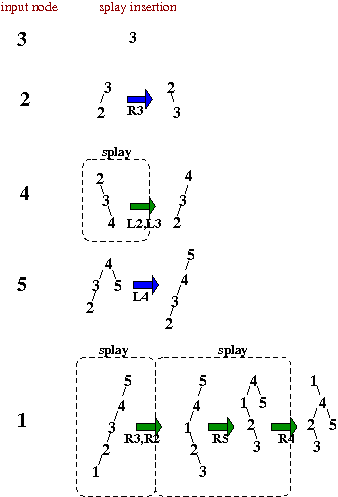
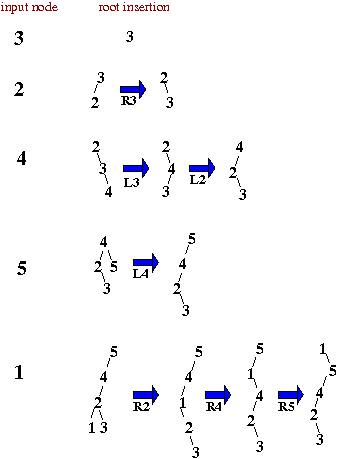
- We have seen 2 types of BST insertion, leaf insertion and root insertion. Assume that we wish to insert the sequence of numbers 3, 2, 4, 5, 1.
- leaf insertion
  - each node is simply added as a leaf
    按照 zigzig，zigzag 来操作
    分别先上后下，先下后上
- root insertion
  - each node is added as a leaf, and then
  - that leaf is promoted to the root position rotating the parent node to the left or right at each step
    就是一个一个移动到 root
Operation splay deletion
- Normally, when an item is deleted in a BST, the node is simply replaced by its DLMD predecessor or DRMD successor.
  
  In splay-deletion, if you wish to delete a node y:
  - delete the node y as normal using either:
    - DRMD: if the predecessor of y is x, and the parent of x is p, then
      - follow the deletion by splaying p
    - DLMD: if the successor node of y is z, and the parent of z is p, then
      - follow the deletion by splaying p
        首先把 DLMD 替换 root，然后再将 DLMD 的 parent 移动到 root
  For example, let us splay-delete item 2 from the following splay tree
  we will use DLMD (DRMD would be similar)
  要删除2节点
  successor为4,4的父节点是5
  把4替换为2节点，作为root
  再把5移动上去
Minimum or maximum of a splay tree
- search for the minimum or maximum item in a BST
- splay the item to the root
- For example, we show below a splay tree after
  1. a search for the minimum and then
  2. a search for the maximum item
in summary

2-3-4 trees
- We note that in each operation:
  - search for an item in a splay tree
    - the found item is splayed
    - if not found, the last accessed item is splayed
  - insertion of an item in a splay tree
    - the new item is splayed
  - deletion of an item in a splay tree
    - the parent of the item that replaces the deleted item is splayed
  - search for the minimum/maximum item in a splay tree
    - the found minimum/maximum is splayed
  A 'splay' of an item involves:
  - zigzig whenever the item is left-left or right-right
  - zigzag whenever the item is left-right or right-left
  - zig whenever the item is the child of the root (so a zigzag or zigzig is not possible)
  Splay Tree Analysis
  - number of comparisons per operation is O(log(n))
  - gives good (amortized) cost overall
  - no guarantee for any individual operation: worst-case behaviour may still be O(N)
  - remember, amortisation means averaging over a large number k operations
- is self-balancing
- is commonly used to implement dictionaries
- has the property that all external nodes are at the same depth
- generalises the concept of a node
  - 3 types of nodes:
    - 2nodes: 1 key, a left link to smaller nodes, a right link to larger nodes (this is the normal node)
      里面只有一个key就是一个字母，类似二叉树，因此只存在大小之分
    - 3nodes: 2 keys, a left link to smaller, a middle link to in-between, and right link to larger
      里面两个字母，小于，中间，大于三部分
    - 4nodes: 3 keys: links to the 4 ranges
      三个字母， M O S 分成四个部分
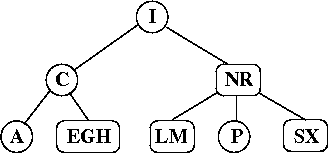
  - In the above example:
    - 'A' is an example of a 2node
    - 'LM' of a 3node
    - 'EGH' of a 4node
    Example of a search, for 'P':
    - start at the root 'I'
    - larger, go right to 'NR'
    - middle, go middle to 'P'
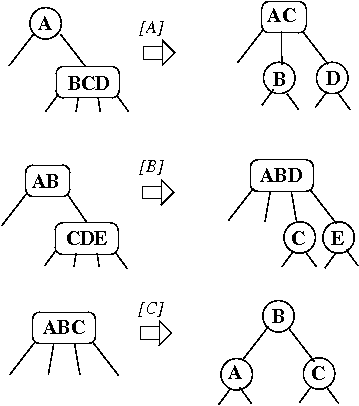
    Examples of insertions:
    - 'B': easy, change node 'A' into 'AB'
    - 'O': easy, change node 'NR' into 'NOR'
    - 'D': the node is full ??
    4node insertions
    - split the 4node分裂4度节点:
      - left key becomes a new 2node 左节点变成一个新的2度节点
      - middle key goes up to parent 中间节点上提到parent里面
      - right node becomes a new 2node 右节点变成一个新的2度节点
    - add new key to one of the new 2nodes, creating a 3node
    Example: insert 'D' in a 2-3-4 tree
    
    This insertion assumes that you can move the 'middle' key up to the parent.
    
    What happens if the parent is a 4node, and its parent is a 4node, and its parent ... ?
    
    Solution, during insertion, on the way down:
    - [A] transform 2node->4node into 3node->(2node+2node)
    - [B] transform 3node->4node into 4node->(2node+2node)
    - [C] if the root is a 4node, transform into 2node->(2node+2node)
    Example:
    
    Basic insertion strategy:
    - because of the transformations (splits) on inserts on the way down, we often end on a 2node or 3node, so easy to insert
    - insert at the bottom:
      - if 2node or 3node, simply include new key
      - if 4node, split the node
        4node需要将中间节点上调，上调后parent又需要上调，以此类推
        相当于递归了
        if the parent is a 4node, this needs to be split
        if the grandparent is a 4node, this needs to be split
        ...
    Results:
    - trees are
      - split from the top down
      - (split and) grow from the bottom up
    - after insertions or deletions, 2-3-4 trees remain in perfect balance
    - What do you notice about these search trees?
    2-3-4 trees are actually implemented using BSTs!