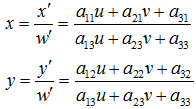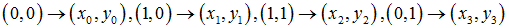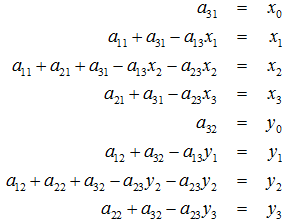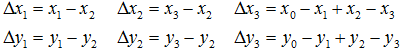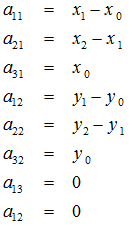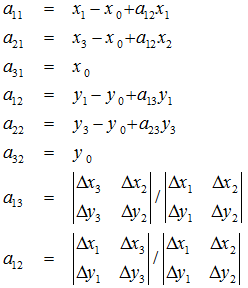透视变换(Perspective Transformation)是将图片投影到一个新的视平面(Viewing Plane)，也称作投影映射(Projective Mapping)。
原理：
通用的变换公式为：
u,v是原始图片左边，对应得到变换后的图片坐标x,y,其中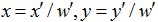
。
变换矩阵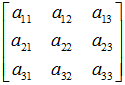
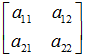
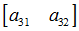
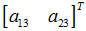
可以拆成4部分，
表示线性变换，比如scaling，shearing和ratotion。
用于平移，
产生透视变换。所以可以理解成仿射等是透视变换的特殊形式。经过透视变换之后的图片通常不是平行四边形（除非映射视平面和原来平面平行的情况）。
重写之前的变换公式可以得到：
所以，已知变换对应的几个点就可以求取变换公式。反之，特定的变换公式也能新的变换后的图片。
变换矩阵的求解：
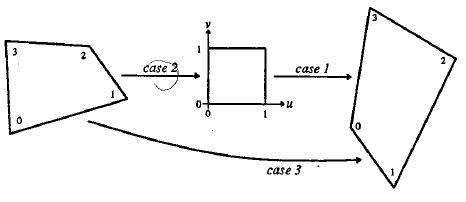
简单的看一个正方形到四边形的变换：
变换的4组对应点可以表示成：
根据变换公式得到：
定义几个辅助变量：
都为0时变换平面与原来是平行的，可以得到：
不为0时，得到：
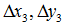
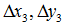
求解出的变换矩阵就可以将一个正方形变换到四边形。反之，四边形变换到正方形也是一样的。
应用：
于是，我们通过两次变换：四边形变换到正方形+正方形变换到四边形就可以将任意一个四边形变换到另一个四边形。
opencv函数：
校正前的四边形定点坐标：
srcTri[0].x = x_lt;
srcTri[0].y = y_lt;
srcTri[1].x = x_rt;
srcTri[1].y = y_rt;
srcTri[2].x = x_lb;
srcTri[2].y = y_lb;
srcTri[3].x = x_rb;
srcTri[3].y = y_rb;
校正后的四边形定点坐标：
dstTri[0].x = x_lt;
dstTri[0].y = y_lt;
dstTri[1].x = x_lt+dist_h;
dstTri[1].y = y_lt;
dstTri[2].x = x_lt;
dstTri[2].y = y_lt+dist_v;
dstTri[3].x = x_lt+dist_h;
dstTri[3].y = y_lt+dist_v;
Mat M=getPerspectiveTransform(srcTri,dstTri);
warpPerspective( src, dst_correct,M,Size(src.cols ,src.rows), INTER_LINEAR, BORDER_CONSTANT,Scalar());
详见原创：http://blog.csdn.net/xiaowei_cqu/article/details/26478135
待解决：w及w'是什么，如何赋值？？如果你知道可以留言告知，方便大家理解吧！来源：CSDN
作者：dingyuanbluesky
链接：https://blog.csdn.net/u012380663/article/details/43272851