二叉搜索树（Binary Search Tree）

二叉搜索树（BST，Binary Search Tree），也称二叉排序树或二叉查找树。

二叉搜索树：一棵二叉树，可以为空；如果不为空，满足以下性质：

非空左子树的所有键值小于其根结点的键值；
非空右子树的所有键值大于其根结点的键值；
左右子树都是二叉搜索树；

Wiki中的定义：

The left subtree of a node contains only nodes with keys less than the node's key.
The right subtree of a node contains only nodes with keys greater than the node's key.
The left and right subtrees each must also be a binary search tree.
Each node can have up to two successor nodes.
There must be no duplicate nodes.
A unique path exists from the root to every other node.

二叉搜索树操作的特别函数：

Position Find（ElementType X，BinTree BST）：从二叉搜索树BST查找元素X，返回其所在结点的地址；

Position FindMin（BinTree BST）：从二叉搜索树BST中查找并返回最小元素所在结点的地址；

Position FindMax（BinTree BST）：从二叉搜索树BST中查找并返回最大元素所在结点的地址；

BinTree Insert（ElementType X，BinTree BST）：把元素X结点插入到二叉搜索树BST中；

BinTree Delete（ElementType X，BinTree BST）：从二叉搜索树BST中删除元素X结点；

【二叉搜索树的查找操作：Find】

1、查找从根结点开始，如果树为空，返回NULL；

2、若搜索树非空，则根结点关键字和X进行比较，并进行不同处理：

如果X小于根结点的键值，只需在左子树中继续搜索；
如果X大于根结点的键值，只需在右子树中继续搜索；
若两者比较结果是相等，搜索完成，返回指向此结点的指针；

递归查找操作：

 1 Position BST_Find(ElementType X, BinTree BST)
 2 {
 3     if(BST == NULL)
 4         return NULL;
 5 
 6     if(X > BST->Data)
 7         return BST_Find(X, BST->Right);
 8     else if(X < BST->Data)
 9         return BST_Find(X, BST->Left);
10     else
11         return BST;
12 }

非递归查找操作：

 1 Postion BST_Find_ext(ElementType X, BinTree BST)
 2 {
 3     while(BST != NULL)
 4     {
 5         if(X > BST->Data)
 6             BST = BST->Right;
 7         else if(X < BST->Data)
 8             BST = BST->Left;
 9         else
10             return BST;
11     }
12     return NULL;
13 }

【二叉搜索树查找最大元素FindMax和最小元素FindMin】

最大元素一定是在树的最右分支的端结点上；

最小元素一定是在树的最左分支的端结点上；

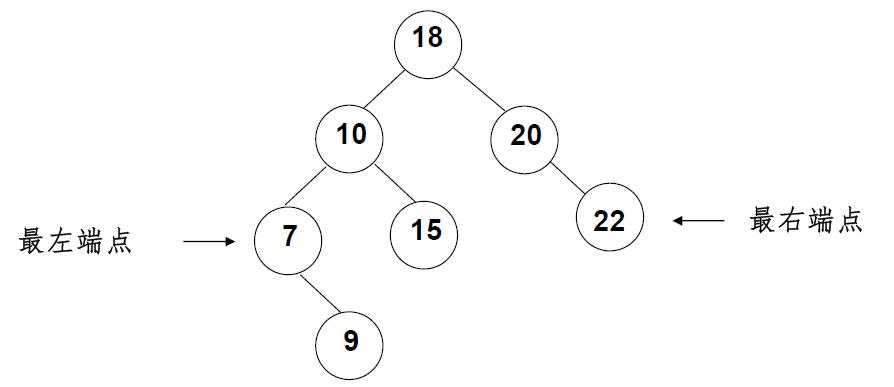
递归查找Min和Max：

 1 Position BST_FindMin(BinTree BST)
 2 {
 3     if(BST == NULL)
 4         return NULL;
 5     else if(BST->Left == NULL)
 6         return BST;
 7     else
 8         return BST_FindMin(BST->Left);
 9 }
10 
11 Position BST_FindMax(BinTree BST)
12 {
13     if(BST == NULL)
14         return NULL;
15     else if(BST->Right == NULL)
16         return BST;
17     else
18         return BST_FindMax(BST->Right);
19 }

非递归查找Min和Max：

 1 Position BST_FindMin_ext(BinTree BST)
 2 {
 3     if(BST != NULL)
 4     {
 5         while(BST->Left != NULL)
 6             BST = BST->Left;
 7     }
 8     return BST;
 9 }
10 
11 Position BST_FindMax_ext(BinTree BST)
12 {
13     if(BST != NULL)
14     {
15         while(BST->Right != NULL)
16             BST = BST->Right;
17     }
18     return BST;
19 }

【二叉搜索树的插入操作：Insert】

 1 BinTree BST_Insert(ElementType X, BinTree BST)
 2 {
 3     if(BST == NULL)
 4     {
 5         // 若原树为空,生成并返回一个结点的二叉搜索树
 6         BST = malloc(sizeof(struct TreeNode));
 7         BST->Data  = X;
 8         BST->Left  = NULL;
 9         BST->Right = NULL;
10     }
11     else
12     {
13         // 开始找要插入元素的位置
14         if(X < BST->Data)
15             BST->Left = BST_Insert(X, BST->Left); // 递归插入左子树
16         else if(X > BST->Data)
17             BST->Right = BST_Insert(X, BST->Right); // 递归插入右子树
18         // else X已经存在,什么都不做
19     }
20     return BST;
21 }

【二叉搜索树的删除操作：Delete】

二叉搜索树的删除操作需要考虑三种情况：

要删除的是叶结点：直接删除，并修改其父结点指针置为NULL；
要删除的结点只有一个孩子结点：将其父结点的指针指向要删除结点的孩子结点；
要删除的结点有左右两棵子树：用另一个结点替代被删除结点，右子树的最小元素或者左子树的最大元素；

 1 BinTree BST_Delete(ElementType X, BinTree BST)
 2 {
 3     Position Tmp;
 4 
 5     if(BST == NULL)
 6     {
 7         printf("warning: xxx\n"); // 未找到要删除的元素
 8     }
 9     else if(X < BST->Data)
10     {
11         BST->Left = BST_Delete(X, BST->Left); // 左子树递归删除
12     }
13     else if(X > BST->Data)
14     {
15         BST->Right = BST_Delete(X, BST->Right); // 右子树递归删除
16     }
17     else
18     {
19         // 被删除结点有左右两个子结点
20         if(BST->Left != NULL && BST->Right != NULL)
21         {
22             // 在右子树中找到最小的元素填充删除结点
23             Tmp = BST_FindMin(BST->Right);
24             BST->Data = Tmp->Data;
25             // 在删除结点的右子树中删除最小元素
26             BST->Right = BST_Delete(BST->Data, BST->Right);
27         }
28         else // 被删除结点有一个或无子结点
29         {
30             Tmp = BST;
31             if(BST->Left == NULL) // 有右孩子或者无子结点
32                 BST = BST->Right;
33             else if(BST->Right == NULL) // 有左孩子或者无子结点
34                 BST = BST->Left;
35             free(Tmp);
36         }
37     }
38     return BST;
39 }

Wiki链接：

Binary_search_tree ：http://en.wikipedia.org/wiki/Binary_search_tree

来源：https://www.cnblogs.com/utank/p/4277072.html

标签

二叉搜索树

bst