第一章第十三题（代数：求解2 × 2线性方程组）(Algebra: solve 2 × 2 linear equations)

*1.13（代数：求解2 × 2线性方程组）可以使用Cramer法则解下面的2 × 2线性方程组，假定ad-bc不为0：

ax + by = e
cx + dy = f

x = (ed-bf)÷(ad-bc)
y = (af-ec)÷(ad-bc)

编写程序，求解以下方程组并显示x和y的值（提示：将公式中的符号替换为数值，从而计算x和y。本练习题可以不运用后面章节的知识而在本章中完成）。

3.4x + 50.2y = 44.5
2.1x + .55y = 5.9

*1.13 (Algebra: solve 2 * 2 linear equations) You can use Cramer’s rule to solve the following 2 * 2 system of linear equation provided that ad – bc is not 0:

ax + by = e
cx + dy = f

x = (ed-bf)÷(ad-bc)
y = (af-ec)÷(ad-bc)

Write a program that solves the following equation and displays the value for x and y: (Hint: replace the symbols in the formula with numbers to compute x and y. This exercise can be done in Chapter 1 without using materials in later chapters.)

3.4x + 50.2y = 44.5
2.1x + .55y = 5.9

下面是参考答案代码:

public class SolveLinearEquation2Times2Question13 {
	public static void main(String[] args) {
	
		final double A,B,C,D,E,F;
		A = 3.4; B = 50.2; C = 2.1;
		D = 0.55; E = 44.5; F = 5.9;
		
		double x,y;
		x = (E * D - B * F) / (A * D - B * C);
		y = (A * F - E * C) / (A * D - B * C);

		System.out.println("X is " + x);
		System.out.println("Y is " + y);
	}
}

运行结果：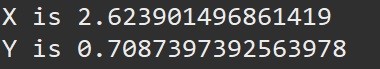

注：编写程序要养成良好习惯
如：1.文件名要用英文，具体一点
2.注释要英文
3.变量命名要具体，不要抽象（如:a,b,c等等），形式要驼峰化
4.整体书写风格要统一（不要这里是驼峰，那里是下划线，这里的逻辑段落空三行，那里相同的逻辑段落空5行等等）

来源：CSDN

作者：xjlovewjh

链接：https://blog.csdn.net/xjlovewjh/article/details/104106909