LaTeX编写分段函数

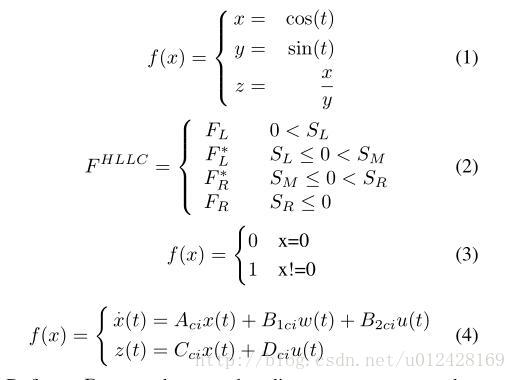
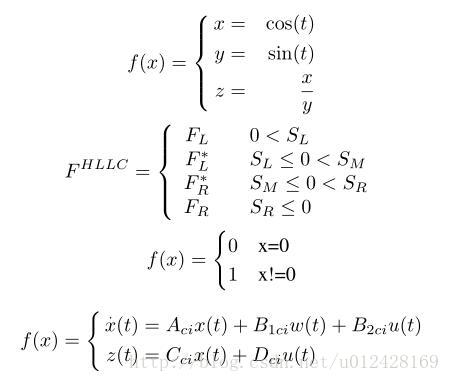如果写成下面这种形式，在论文中你的公式会自动排序。

\begin{equation}
f(x)=\left\{
\begin{aligned}
x & = & \cos(t) \\
y & = & \sin(t) \\
z & = & \frac xy
\end{aligned}
\right.
\end{equation}

\begin{equation}
F^{HLLC}=\left\{
\begin{array}{rcl}
F_L & & {0 < S_L}\\
F^*_L & & {S_L \leq 0 < S_M}\\
F^*_R & & {S_M \leq 0 < S_R}\\
F_R & & {S_R \leq 0}
\end{array} \right.
\end{equation}

\begin{equation}
f(x)=
\begin{cases}
0& \text{x=0}\\
1& \text{x!=0}
\end{cases}
\end{equation}

\begin{equation}
\left\{
\begin{aligned}
\overset{.}x(t) &=A_{ci}x(t)+B_{1ci}w(t)+B_{2ci}u(t) \\
z(t) &=C_{ci}x(t)+D_{ci}u(t) \\
\end{aligned}
\right.
\end{equation}

这里写图片描述
而如果写成下面这种形式，就只是行内公式，不具有自动排序功能。

$$ f(x)=\left\{
\begin{aligned}
x & = & \cos(t) \\
y & = & \sin(t) \\
z & = & \frac xy
\end{aligned}
\right.
$$

$$ F^{HLLC}=\left\{
\begin{array}{rcl}
F_L & & {0 < S_L}\\
F^*_L & & {S_L \leq 0 < S_M}\\
F^*_R & & {S_M \leq 0 < S_R}\\
F_R & & {S_R \leq 0}
\end{array} \right. $$

$$f(x)=
\begin{cases}
0& \text{x=0}\\
1& \text{x!=0}
\end{cases}$$

$$
f(x)=\left\{
\begin{aligned}
\overset{.}x(t) &=A_{ci}x(t)+B_{1ci}w(t)+B_{2ci}u(t) \\
z(t) &=C_{ci}x(t)+D_{ci}u(t) \\
\end{aligned}
\right.
$$