题目描述:这里
思路:
一、部分分算法
- 对于
 的数据,用暴力解决即可,时间复杂度
的数据,用暴力解决即可,时间复杂度
- 对于另外
 的数据(所有木棍长度相等),考虑用组合数学,答案为
的数据(所有木棍长度相等),考虑用组合数学,答案为
二、正解
我们考虑对整个序列进行桶排序。
我们设每个数出现的次数为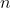 。
。
- 对于所有
 ≥
≥ 的数,加上比它小的所有数出现的次数,并加上这个数
的数,加上比它小的所有数出现的次数,并加上这个数 至这个数
至这个数 中所有数出现的个数。
中所有数出现的个数。 - 特别的,对于所有
 ≥
≥ 的数,需要再次加上
的数,需要再次加上 。
。
但是,我们会发现这依然过不了(TLE了一个点)。
我们再次仔细观察正解的统计方式第一条,发现这可以用前缀和优化。
于是,这道题就做完了。
下面附上代码:
#include <bits/stdc++.h>
using namespace std;
template < typename T > void read(T &x)
{
int f = 1;x = 0;char c = getchar();
for (;!isdigit(c);c = getchar()) if (c == '-') f = -f;
for (; isdigit(c);c = getchar()) x = x * 10 + c - '0';
x *= f;
}
const long long mod = 998244353;
int a[200005];
long long bin[200005];
long long sum[200005];
int main()
{
//freopen(".in", "r", stdin);
//freopen(".out", "w", stdout);
int n, binmax = INT_MIN;
long long ans = 0;
read(n);
for(int i = 1;i <= n;i++)
{
read(a[i]);
bin[a[i]]++;
binmax = max(binmax, a[i]);
}
int t = a[1];
int flag = 1;
for(int i = 2;i <= n;i++)
if(a[i] != t)
{
flag = 0;
break;
}
if(flag)
{
long long ans = 1;
for(int i = n;i > n - 3;i--)
{
ans *= i;
}
cout << (ans / 6) % mod << endl;
return 0;
}
if(n <= 200)
{
for(int i = 1;i <= n;i++)
for(int j = i + 1;j <= n;j++)
for(int k = j + 1;k <= n;k++)
if((a[i] == a[j] || a[i] == a[k] || a[j] == a[k]) && (a[i] + a[j] > a[k]) && a[i] + a[k] > a[j] && a[j] + a[k] > a[i])
ans++;
cout << ans % mod << endl;
return 0;
}
sum[0] = 0;
for(int i = 1;i <= binmax;i++)
sum[i] = bin[i] + sum[i - 1];
long long front = 0;
for(int i = 1;i <= binmax;++i)
if(bin[i])
{
if(bin[i] >= 3)
{
long long ansj = 1;
for(int j = bin[i];j > bin[i] - 3;--j)
ansj *= j;
ans += ansj / 6;
ans %= mod;
}
if(bin[i] >= 2)
{
long long tx = bin[i] * (bin[i] - 1) / 2;
ans += front * tx;
ans %= mod;
long long up = min(i * 2, binmax + 1) - 1;
long long ty = sum[up] - sum[i];
ans += ty * tx;
ans %= mod;
}
front += bin[i];
}
cout << ans << endl;
return 0;
}
来源:oschina
链接:https://my.oschina.net/u/4385631/blog/4437751
 的数据,用暴力解决即可,时间复杂度
的数据,用暴力解决即可,时间复杂度
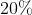 的数据(所有木棍长度相等),考虑用组合数学,答案为
的数据(所有木棍长度相等),考虑用组合数学,答案为
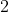 的数,加上比它小的所有数出现的次数,并加上这个数
的数,加上比它小的所有数出现的次数,并加上这个数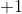 至这个数
至这个数 中所有数出现的个数。
中所有数出现的个数。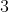 的数,需要再次加上
的数,需要再次加上