基本概念
ROC全称是“受试者工作特征”(Receiver Operating Characteristic)曲线。ROC曲线的纵轴是“真正率”(True Positive Rate, TPR),横轴是“假正例率”(False Positive Rate, FPR)。
TPR=TP/(TP+FN)
FPR= FP/(TN+FP)

进行学习器的比较时,若一个学习器的ROC曲线被另一个学习器的曲线完全“包住”,则可以断言后者的性能优于前者;若两个学习器的ROC曲线发生交叉,则难以一般性地断言两者孰优孰劣。此时较为合理的判据是比较ROC曲面下的面积,即AUC(Area Under ROC Curve)。
示例演示
我们看看sklearn的官方例子:Receiver Operating Characteristic (ROC) with cross validation。
"""
=============================================================
Receiver Operating Characteristic (ROC) with cross validation
=============================================================
Example of Receiver Operating Characteristic (ROC) metric to evaluate
classifier output quality using cross-validation.
ROC curves typically feature true positive rate on the Y axis, and false
positive rate on the X axis. This means that the top left corner of the plot is
the "ideal" point - a false positive rate of zero, and a true positive rate of
one. This is not very realistic, but it does mean that a larger area under the
curve (AUC) is usually better.
The "steepness" of ROC curves is also important, since it is ideal to maximize
the true positive rate while minimizing the false positive rate.
This example shows the ROC response of different datasets, created from K-fold
cross-validation. Taking all of these curves, it is possible to calculate the
mean area under curve, and see the variance of the curve when the
training set is split into different subsets. This roughly shows how the
classifier output is affected by changes in the training data, and how
different the splits generated by K-fold cross-validation are from one another.
.. note::
See also :func:`sklearn.metrics.roc_auc_score`,
:func:`sklearn.model_selection.cross_val_score`,
:ref:`sphx_glr_auto_examples_model_selection_plot_roc.py`,
"""
print(__doc__)
import numpy as np
from scipy import interp
import matplotlib.pyplot as plt
from sklearn import svm, datasets
from sklearn.metrics import auc
from sklearn.metrics import plot_roc_curve
from sklearn.model_selection import StratifiedKFold
# #############################################################################
# Data IO and generation
# Import some data to play with
iris = datasets.load_iris()
X = iris.data
y = iris.target
X, y = X[y != 2], y[y != 2]
n_samples, n_features = X.shape
# Add noisy features
random_state = np.random.RandomState(0)
X = np.c_[X, random_state.randn(n_samples, 200 * n_features)]
# #############################################################################
# Classification and ROC analysis
# Run classifier with cross-validation and plot ROC curves
cv = StratifiedKFold(n_splits=6)
classifier = svm.SVC(kernel='linear', probability=True,
random_state=random_state)
tprs = []
aucs = []
mean_fpr = np.linspace(0, 1, 100)
fig, ax = plt.subplots()
for i, (train, test) in enumerate(cv.split(X, y)):
classifier.fit(X[train], y[train])
viz = plot_roc_curve(classifier, X[test], y[test],
name='ROC fold {}'.format(i),
alpha=0.3, lw=1, ax=ax)
interp_tpr = interp(mean_fpr, viz.fpr, viz.tpr)
interp_tpr[0] = 0.0
tprs.append(interp_tpr)
aucs.append(viz.roc_auc)
ax.plot([0, 1], [0, 1], linestyle='--', lw=2, color='r',
label='Chance', alpha=.8)
mean_tpr = np.mean(tprs, axis=0)
mean_tpr[-1] = 1.0
mean_auc = auc(mean_fpr, mean_tpr)
std_auc = np.std(aucs)
ax.plot(mean_fpr, mean_tpr, color='b',
label=r'Mean ROC (AUC = %0.2f $\pm$ %0.2f)' % (mean_auc, std_auc),
lw=2, alpha=.8)
std_tpr = np.std(tprs, axis=0)
tprs_upper = np.minimum(mean_tpr + std_tpr, 1)
tprs_lower = np.maximum(mean_tpr - std_tpr, 0)
ax.fill_between(mean_fpr, tprs_lower, tprs_upper, color='grey', alpha=.2,
label=r'$\pm$ 1 std. dev.')
ax.set(xlim=[-0.05, 1.05], ylim=[-0.05, 1.05],
title="Receiver operating characteristic example")
ax.legend(loc="lower right")
plt.show()
运行结果
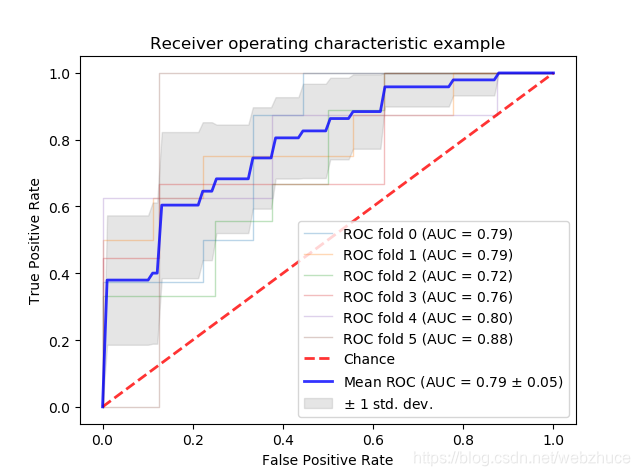
来源:CSDN
作者:阿兵-AI医疗
链接:https://blog.csdn.net/webzhuce/article/details/104220772